The parabolic segment
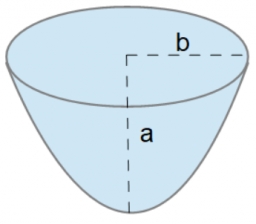
The parabolic segment has a base a = 4 cm and a height v = 6 cm. Calculate the volume of the body that results from the rotation of this segment
a) around its base
b) around its axis.
a) around its base
b) around its axis.
Correct answer:
You need to know the following knowledge to solve this word math problem:
- algebra
- expression of a variable from the formula
- arithmetic
- square (second power, quadratic)
- planimetrics
- area of a shape
- basic functions
- functions
- integral
Units of physical quantities:
Grade of the word problem:
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- A park on map
 A park has an area of ⅙ mi². On a map, the park has an area of 1 ¼ cm². On the map, how many square centimeters represent 1 mi²?
A park has an area of ⅙ mi². On a map, the park has an area of 1 ¼ cm². On the map, how many square centimeters represent 1 mi²? - The rectangle 7
 The rectangle shown has a length (l) of 8 and a width (w) of 6.2. A second rectangle has a length of l + 2.5 and a width of w - 1.4. Find the area of the SECOND rectangle.
The rectangle shown has a length (l) of 8 and a width (w) of 6.2. A second rectangle has a length of l + 2.5 and a width of w - 1.4. Find the area of the SECOND rectangle. - A room
 A room is 8 2/3 m by 5 1/4 m. What is the area of the room?
A room is 8 2/3 m by 5 1/4 m. What is the area of the room? - Ice cream company
 An ice cream company is going to open new stores in Los Angeles, New York, and Orlando. The monthly cost for a square foot of rental space in each city is as follows. town; cost/ft² Los Angeles ; $5.25 New York ; $7.25 Orlando ; $4.25 The store in Los Ang
An ice cream company is going to open new stores in Los Angeles, New York, and Orlando. The monthly cost for a square foot of rental space in each city is as follows. town; cost/ft² Los Angeles ; $5.25 New York ; $7.25 Orlando ; $4.25 The store in Los Ang
- The capacitor
 The parallel square plates of a 4.5µF Teflon capacitor are 3.2 mm apart. What is the area of the plate?
The parallel square plates of a 4.5µF Teflon capacitor are 3.2 mm apart. What is the area of the plate? - A model
 A model of a house is built on a base that measures 7 3/4 inches wide and 9 1/5 inches long. What is the area of the model house based?
A model of a house is built on a base that measures 7 3/4 inches wide and 9 1/5 inches long. What is the area of the model house based? - A rectangle 13
 A rectangle has an area of 15 square inches. What is the missing side length if one side length is 1 2/3 inches wide?
A rectangle has an area of 15 square inches. What is the missing side length if one side length is 1 2/3 inches wide? - Trim the bushes
 Mason is always helping him to trim the bushes. The area A had a length of 14 m and a breadth of 7 m, and if we are going to combine A and B, the equal is 215 m². The question is: what is the area of B?
Mason is always helping him to trim the bushes. The area A had a length of 14 m and a breadth of 7 m, and if we are going to combine A and B, the equal is 215 m². The question is: what is the area of B? - Harold
 Harold made a rectangular dog run in his backyard. The area of the dog run is 96 square feet. What are three different possible dimensions of dog run?
Harold made a rectangular dog run in his backyard. The area of the dog run is 96 square feet. What are three different possible dimensions of dog run?
- The triangle 6
 The triangle has an area of 7 ⅞ cm² and a base of 5 ¼ cm. What is the length of h? Explain your reasoning.
The triangle has an area of 7 ⅞ cm² and a base of 5 ¼ cm. What is the length of h? Explain your reasoning. - A concert venue
 The floor of a concert venue is 100 3/4 feet by 75 1/2 feet. What is the area of the floor?
The floor of a concert venue is 100 3/4 feet by 75 1/2 feet. What is the area of the floor? - A farmer 9
 A farmer has 3 hectares of an orchard. ½ of the land is occupied by apples, ⅙ of the remainder is occupied by lemon trees, and tree tomatoes occupy the rest of it. Find the fraction of the land occupied by tree tomatoes.
A farmer has 3 hectares of an orchard. ½ of the land is occupied by apples, ⅙ of the remainder is occupied by lemon trees, and tree tomatoes occupy the rest of it. Find the fraction of the land occupied by tree tomatoes. - The bedroom
 The area of the rectangular bedroom is 136 5/8 square feet. If its width is 12 3/4 feet, find its length in simplest form.
The area of the rectangular bedroom is 136 5/8 square feet. If its width is 12 3/4 feet, find its length in simplest form. - Nisha
 Nisha likes to make small rectangular paintings of cats. One of her paintings has an area of 33cm squared and a width of 7 1/2 centimeters. What is the height of this painting?
Nisha likes to make small rectangular paintings of cats. One of her paintings has an area of 33cm squared and a width of 7 1/2 centimeters. What is the height of this painting?
- Yd2 to ft2 unit conversion
 If a room requires 25.4 square yards of carpeting, what is the area of the floor in units of ft2? (3 ft. = 1 yd.)
If a room requires 25.4 square yards of carpeting, what is the area of the floor in units of ft2? (3 ft. = 1 yd.) - Panting
 If the base of the triangle is 2 3/4 meters & and the altitude is 3 2/3 meters, then what is the cost of panting on both sides at ₹3 1/2 per sq.m.?
If the base of the triangle is 2 3/4 meters & and the altitude is 3 2/3 meters, then what is the cost of panting on both sides at ₹3 1/2 per sq.m.? - Sq feet converions
 What is the area in square feet of a room that is 10' 3" x 16' 9"?
What is the area in square feet of a room that is 10' 3" x 16' 9"?
