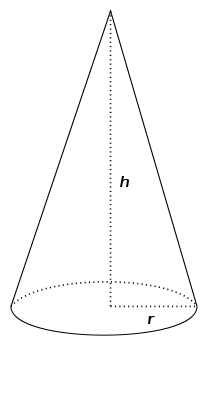
Volume of the cone
Find the volume of the cone with the base radius r and the height v.
a) r = 6 cm, v = 8 cm
b) r = 0,9 m, v = 2,3 m
c) r = 1,4 dm, v = 30 dm
a) r = 6 cm, v = 8 cm
b) r = 0,9 m, v = 2,3 m
c) r = 1,4 dm, v = 30 dm
Correct answer:
Tips for related online calculators
Tip: Our volume units converter will help you convert volume units.
You need to know the following knowledge to solve this word math problem:
Units of physical quantities:
Grade of the word problem:
Related math problems and questions:
- Geometric Solid
 A concrete pipe is 1.0 m long. Its inside and outside diameters are 60 cm and 75 cm, respectively. What is the volume of the concrete (cubic meters) needed to make 25 pcs of pipe?
A concrete pipe is 1.0 m long. Its inside and outside diameters are 60 cm and 75 cm, respectively. What is the volume of the concrete (cubic meters) needed to make 25 pcs of pipe? - Understanding 81807
 The aquarium is 0.7m long and 25cm wide. The battery is deep if it can hold no more than 87.5 liters of water. I need help understanding how to calculate this.
The aquarium is 0.7m long and 25cm wide. The battery is deep if it can hold no more than 87.5 liters of water. I need help understanding how to calculate this. - Dimensions 81805
 The soap has the shape of a cuboid with dimensions of 6 cm, 4 cm, and 2 cm. Katy used it for a week and all the dimensions of the soap shrunk by half. How long will her soap last?
The soap has the shape of a cuboid with dimensions of 6 cm, 4 cm, and 2 cm. Katy used it for a week and all the dimensions of the soap shrunk by half. How long will her soap last? - Create 6
 Create a new drink can that will double the volume of the original can used. Dimensions of the can: 6cm diameter and 10cm height. You are to double the volume with the least amount of additional aluminum needed for the new can. There are 3 options : Optio
Create a new drink can that will double the volume of the original can used. Dimensions of the can: 6cm diameter and 10cm height. You are to double the volume with the least amount of additional aluminum needed for the new can. There are 3 options : Optio - The volume 7
 The volume of a cylindrical can is 75.36 in³. The diameter is 4 inches. Find the height. Use 3.14 as an estimation for pi.
The volume of a cylindrical can is 75.36 in³. The diameter is 4 inches. Find the height. Use 3.14 as an estimation for pi. - 6 Pipes
 6 Pipes are required to fill a tank in 1 hour 20 minutes. How long will it take if only five pipes of the same type are used? Give your answer in hours and minutes.
6 Pipes are required to fill a tank in 1 hour 20 minutes. How long will it take if only five pipes of the same type are used? Give your answer in hours and minutes. - A bus 3
 A bus has a petrol tank in the shape of a cylinder. The cylinder is 125 cm long and has a diameter of 42 cm. (i) How much will a full tank of petrol cost at the rate of 5.23 per liter? (ii) If the bus uses petrol at 12.5 liters for every 50 km, how far ca
A bus has a petrol tank in the shape of a cylinder. The cylinder is 125 cm long and has a diameter of 42 cm. (i) How much will a full tank of petrol cost at the rate of 5.23 per liter? (ii) If the bus uses petrol at 12.5 liters for every 50 km, how far ca - Joanne 2
 Joanne bought five fish. For fish to have enough space, they must have at least 30 liters of water. You know that the length is 5 dm and the width is 3 dm. Calculate the minimum height of the aquarium.
Joanne bought five fish. For fish to have enough space, they must have at least 30 liters of water. You know that the length is 5 dm and the width is 3 dm. Calculate the minimum height of the aquarium. - Cylindrical 71714
 How many hectoliters of water are in a cylindrical water tank with a base diameter of 3 meters and a depth of 60 cm?
How many hectoliters of water are in a cylindrical water tank with a base diameter of 3 meters and a depth of 60 cm? - Quadrilateral 70294
 The edge lengths of a quadrilateral prism are in the ratio a: b: c = 2:4:5. The surface of the prism is 57 cm². Calculate the volume.
The edge lengths of a quadrilateral prism are in the ratio a: b: c = 2:4:5. The surface of the prism is 57 cm². Calculate the volume. - Right-angled trapezoid
 A right-angled trapezoid with the measure of the acute angle of 50° is given. The lengths of its bases are 4 and 6 units. The volume of the solid obtained by rotation of the given trapezoid about the longer base is:
A right-angled trapezoid with the measure of the acute angle of 50° is given. The lengths of its bases are 4 and 6 units. The volume of the solid obtained by rotation of the given trapezoid about the longer base is: - Pool model
 The 1:500 scale pool model has internal dimensions of 15 cm, 10 cm, and 2.5 mm. Calculate how many hectoliters of water will be needed to fill a pool that will build according to this model.
The 1:500 scale pool model has internal dimensions of 15 cm, 10 cm, and 2.5 mm. Calculate how many hectoliters of water will be needed to fill a pool that will build according to this model. - Determine 5
 Determine the volume of an aquarium with these measurements: length = 78 cm ; width = 6 cm ; height = 43 cm using the formula V=lwh.
Determine the volume of an aquarium with these measurements: length = 78 cm ; width = 6 cm ; height = 43 cm using the formula V=lwh. - Pipe thickness
 The outer circumference of the tube is 32cm. Its length is 60cm, density 8.5g/cm³ and weight 9.495kg. Calculate the wall thickness of the pipe.
The outer circumference of the tube is 32cm. Its length is 60cm, density 8.5g/cm³ and weight 9.495kg. Calculate the wall thickness of the pipe. - Prism - right isosceles
 Find the volume and surface of a prism with a height of 120 mm, the base of which is a right isosceles triangle with a leg length of 5 cm.
Find the volume and surface of a prism with a height of 120 mm, the base of which is a right isosceles triangle with a leg length of 5 cm. - Sphere volume formula
 If V=4/3 π r³, find the value of V when r = 7, the value of r when V=113 1/7
If V=4/3 π r³, find the value of V when r = 7, the value of r when V=113 1/7 - Side wall planes
 Find the volume and surface of a cuboid whose side c is 30 cm long and the body diagonal forms angles of 24°20' and 45°30' with the planes of the side walls.
Find the volume and surface of a cuboid whose side c is 30 cm long and the body diagonal forms angles of 24°20' and 45°30' with the planes of the side walls.
