Area - math word problems - page 74 of 157
Number of problems found: 3128
- Sheets into container
 How many sheets need to be closed on the block-shaped container above), which is 4 m wide, 250 cm long, and 35 dm high? How many liters of water can fit in it?
How many sheets need to be closed on the block-shaped container above), which is 4 m wide, 250 cm long, and 35 dm high? How many liters of water can fit in it? - Perpendicular 21433
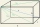 Calculate the height of the vertical prism with the rectangle's base if the dimensions of the edges of the floor are a = 12 dm, b = 50 mm, and the prism's volume V = 0.6 l.
Calculate the height of the vertical prism with the rectangle's base if the dimensions of the edges of the floor are a = 12 dm, b = 50 mm, and the prism's volume V = 0.6 l. - Magnification 21383
 When we increased the circle's radius by 2 cm, its area increased by 40π cm². Determine the radius of the circle before magnification.
When we increased the circle's radius by 2 cm, its area increased by 40π cm². Determine the radius of the circle before magnification. - Rectangular 21353
 The rectangular plot has an area of 480 m². One of its dimensions is 30 m. Calculate the second dimension of the plot.
The rectangular plot has an area of 480 m². One of its dimensions is 30 m. Calculate the second dimension of the plot. - Tiles
 The pupils of the building school have to calculate how many roof tiles they will need to cover the roof of the house in the shape of two rectangles measuring 10 x 7.2 m. One roof tile covers a rectangular area of 22 cm x 32 cm. How many tiles will they n
The pupils of the building school have to calculate how many roof tiles they will need to cover the roof of the house in the shape of two rectangles measuring 10 x 7.2 m. One roof tile covers a rectangular area of 22 cm x 32 cm. How many tiles will they n - Height to the base
 The triangle area is 35 cm². The size of the base is 10 cm. Find the length of height to the base.
The triangle area is 35 cm². The size of the base is 10 cm. Find the length of height to the base. - Circular dragon
 The dragon has the shape of a circular section with a central angle of 40° and a radius of 35 cm. How much paper do we need to make if 10% is added to the curved ones?
The dragon has the shape of a circular section with a central angle of 40° and a radius of 35 cm. How much paper do we need to make if 10% is added to the curved ones? - Calculate S,V
 Calculate the volume and surface area of the sphere if given r = 36mm.
Calculate the volume and surface area of the sphere if given r = 36mm. - Dimensions 21153
 The sheet of paper has the dimensions a = 28 cm and b = 8 cm. How many 4 cm squares can we cut from the paper? (draw a picture)
The sheet of paper has the dimensions a = 28 cm and b = 8 cm. How many 4 cm squares can we cut from the paper? (draw a picture) - Corresponding height
 Calculate the height corresponding to the side of the parallelogram ABCD if the side is 7.4 cm long and the area of the parallelogram is 33.3 cm2
Calculate the height corresponding to the side of the parallelogram ABCD if the side is 7.4 cm long and the area of the parallelogram is 33.3 cm2 - An equilateral
 An equilateral triangle is inscribed in a square of side 1 unit long so that it has one common vertex with the square. What is the area of the inscribed triangle?
An equilateral triangle is inscribed in a square of side 1 unit long so that it has one common vertex with the square. What is the area of the inscribed triangle? - Circumference 20933
 Calculates the circumference of a circle if its area is S = 2119.5 cm².
Calculates the circumference of a circle if its area is S = 2119.5 cm². - Calculate 20893
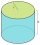 The volume of the cylinder is 193 cm³, and the radius of its base is 6.4 cm. Calculate the height and surface of the cylinder to 1 decimal place.
The volume of the cylinder is 193 cm³, and the radius of its base is 6.4 cm. Calculate the height and surface of the cylinder to 1 decimal place. - Hectares of forest
 Twelve workers plant 24 ha of forest in 6 days. How many days will 15 people and 12 people plant in the same area?
Twelve workers plant 24 ha of forest in 6 days. How many days will 15 people and 12 people plant in the same area? - wheat 20833
 Mr. Vávra sows wheat and rye for a total of 28 ha. Rye sows two and a half times the area of wheat. How many ha sow wheat and how much rye?
Mr. Vávra sows wheat and rye for a total of 28 ha. Rye sows two and a half times the area of wheat. How many ha sow wheat and how much rye? - Material consumption
 The sphere-shaped reservoir has a volume of 282 hl. Calculate the material consumption in m² for its production, assuming 8% for joints and waste, and round the final result to the nearest integers.
The sphere-shaped reservoir has a volume of 282 hl. Calculate the material consumption in m² for its production, assuming 8% for joints and waste, and round the final result to the nearest integers. - The playground
 The playground is a square with a side of 64 m. It is fenced on three sides. What is the playground area, and how long is the fence?
The playground is a square with a side of 64 m. It is fenced on three sides. What is the playground area, and how long is the fence? - The regular
 The regular quadrilateral pyramid has a volume of 24 dm³ and a height of 45 cm. Calculate its surface.
The regular quadrilateral pyramid has a volume of 24 dm³ and a height of 45 cm. Calculate its surface. - Surface 20673
 Find the surface of the cube with an edge of 7.2 cm.
Find the surface of the cube with an edge of 7.2 cm. - Calculate 20643
 Calculate the area and perimeter of the building plot in the shape of an isosceles trapezoid with a base of 120 m, 95 m, and a height of 50 m.
Calculate the area and perimeter of the building plot in the shape of an isosceles trapezoid with a base of 120 m, 95 m, and a height of 50 m.
Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
