Fractions + square (second power, quadratic) - practice problems - page 2 of 8
Number of problems found: 147
- Diophantus
We know little about this Greek mathematician from Alexandria, except that he lived around the 3rd century A.D. Thanks to an admirer who described his life through an algebraic riddle, we know at least something about it. Diophantus's youth lasted 1/6 of - Express value
Given that p = √(mx/t-t² x) Make x the subject If m = 7, p = -3 and t = 4, find the value of x - Fraction unknowns
Divide fractions with unknowns: Fraction 1: The quantity x squared plus 6 times x plus 9 over the quantity x minus 1. Fraction 2: the quantity x squared minus 9 over the quantity x squared minus 2 times x plus 1. Find Fraction 1 over Fraction 2. - Mr. Happy
Mr. Happy planted 36.6 meters of square garden grass; It's a third of the garden, more than half of the garden. What is the square area of this garden?
- The expression
What is the value of the expression ((6²+60))/(23) - The plot
Mr. Duma recently inherited a rectangular plot, part of the estate left by his late father. The plot with the following dimensions: Length=2x+1; Width=x-1. Determine the formula, in terms of x, that best describes the area of the rectangular plot. He has - Tree planting
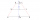The trapezoidal garden has bases 44 m and 16 m long. Their distance is 25 m. How many square meters of its area will remain for tree planting if we use 1/5 of the entire site to construct a cottage, backyard, and road? Is it possible to find the fence len - Circular 72244
How many kg of grass seed must be bought to start a lawn in the shape of a circular section with a radius of r= 15 m and a central angle of 45 degrees if 1 g of grass seed is used per 1 dm of the square area? - Magic belt
The magic rectangular belt has the property that whenever its owner wants something, the length of the belt is reduced to 1/2 and the width to 1/3. After three such wishes, the belt had an area of 4 cm². What was its original length if the original width
- Kilograms 48761
Matthew paints his room. He paints square walls. He painted a wall with a side of 3 m and consumed 5 kg of paint. How many kilograms of paint will he need on a wall with a side length of 6 m? - Mohammed
Mohammed grew a garden last year. His plot of land was 6 3/4 feet by 10 feet. This year, he wants to have twice as much area as his garden. How much area will the new garden take up? - Undeveloped 71924 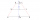The Doležals will build a house. Their plot is a trapezoid with bases 42m and 18m long, and the distance between the bases is 23m. According to the project, the house will have a built-up area of 146 m². How many square meters of land will remain undeve
- Clarissa 2
Clarissa is cutting construction paper into rectangles for a project. She needs to cut one 12 inches x 15 1/3 inches rectangle. She needs to cut another rectangle 10 1/4 inches by 10 1/3 inches. How many Total square inches of construction paper does Clar - Measuring 39011
Miška wants to buy wrapping paper for a gift for her mother. How many euros will he pay if he wants to buy paper measuring 9 quarters of a meter and 8-fifths of a meter, and one square meter of wrapping paper costs 40 cents?
- Garden
The rectangular garden has dimensions of 27 m and 30 m. Peter and Katka split it in a ratio of 4:5. How many square meters did Katkin measure part of the garden? - Hamster cage
Ryan keeps his hamster cage on his dresser. The area of the top of Ryan's dresser is 1 2/3 as large as the area of the bottom of his hamster cage. The area of the dresser top is 960 square inches. How many square inches of his dresser top are not covered - Garden
The garden is rectangular and has lengths of 25 and 40 meters. It has been expanded so that each size increased by one-fifth. How many square meters increased its acreage? - Smaller 81015
Divide the area S=153 m² of the square garden in a ratio of 2:7. What part of the garden does the smaller part occupy? - Connecting lines
They are given a square ABCD. The points EFGH are the midpoints of its sides. What part of the area of the square ABCD is the area of the square created in its center by connecting the points AF, BG, CH, and DE?
Alexandra made a rectangular quilt that measured 3 1/4. 2 3/4 feet in width. What is the area of the quilt in square feet? Write an equation to solve.Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
