Line segment - practice problems
Direction: Solve each problem carefully and show your solution in each item.Number of problems found: 174
- Triangles - segments
How many triangles can be formed with segments measuring one and 2/3 mm one 3/4 mm and 2 1/2 mm - Angle and slope
Find the angle between the x-axis and the line joining the points (3, -1) and (4,-2) . - Two points
M and N are two points on the X-axis and Y-axis, respectively. Point P (3, 2) divides the line segment MN in a ratio of 2:3. Find: (i) the coordinates of M and N (ii) slope of the line MN. - Freezing A certain freezing process requires that room temperature be lowered from 40°C at the rate of 5°C per hour.What will be the room temperature 12 hours after the process begins?
- LS and y-axis intersection In what ratio is the line segment joining P (5, 3) and Q (–5, 3) divided by the y-axis? Also, find the coordinates of the intersection point.
- The co-ordinates
The co-ordinates of the point P dividing the line segment joining the points A (1,3) and B (4,6) internally in the ratio 2:1 are - Midpoint 11 Consider the following line segment - start point A=(-4,1), endpoint B=(4,-1). Find the midpoint. Please show your work.
- PQR - Euclid
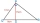Find the length of line segment PR - leg of the right triangle PQR. PQ=17 cm PS=15 cm QS=8 cm; Point S is the height touch point with a hypotenuse of the RQ. - Four points
There are 4 nonlinear points. How many triangles can form by joining them?
- Line segments
There are three line segments on the line: the length of MN = 3 1/2, the length of NO= 2 3/4, and the length of OP=1 2/3. Find the length of line segment MP. Write your answer as a mixed number. - Collinear lines Points A, B, and C are collinear, and B lies between A and C. If AC = 48, AB = 2x + 2, and BC = 3x + 6, what is BC?
- The endpoints
The endpoints of a segment are (-6,1) and (10,11). What are the coordinates of its midpoint? - There 35
There are three points on a straight line: A, BC. If CD = 8x, DE = 3, and CE = x + 10, what is CD? Simplify your answer and write it as a proper fraction, mixed number, or integer. - Line equation: Line equation: y-3=8/9(x-5) Solve for slope
- The volume 8
The volume of a right regular hexagonal prism is 187.2 cubic millimeters. The line segment that has a length of 2.6 millimeters begins at the center of the hexagon and ends at one side of the hexagon. 3 mm base. Find the height. - The slope 2
What is the slope of the line that passes through the points (-4, -7) and (-2,-19)? Write your answer in the simplest form. - A 100-inch
A 100-inch stick is to be divided into four using the ratio 2: 5: 7: 11. How long is the longest piece? - Line segment
Find the length of the line joining points A(-4,8) and B(-1,4). - Midpoint between conjugate
Find the midpoint between two roots: 2+3.464i and 2 - 3.464i
Do you have unsolved problem that you need help? Ask a question, and we will try to solve it. Solving math problems.
