Powers
Is it true for any number a,b,c,d equality:?
(a−b−c−d)6=(d+c+b−a)6
Result
(a−b−c−d)6=(d+c+b−a)6
Result
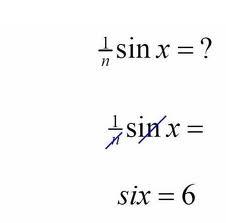
You need to know the following knowledge to solve this word math problem:
Grade of the word problem:
Related math problems and questions:
- Trigonometry
Is true equality? sin(x +13 π)= sin(x) - Two numbers 6
Fill two natural numbers a, b: 7 + blank- blank = 5 - Expression
If it is true that (l + 15 w)/(w) = 6, then the value of the expression (13 l)/(12 w) is: - Primes 2
For what primes p,q,r is true: p²-(q+r)²=647 - Assignment 68484
Write the assignment in a fraction. Fill in the number in the box so that the equality applies: (Write the result as a fraction in the base form.) 3 · ___ + 1/4 = 5/8 - For what
For what value of x is -5x+8=-6 a true statement? Write your answer as a decimal. - Brackets 2
Add parenthesis to make true: 5-2×6-4+2=5 - Height
Is it true that the height is less or equal to half of the hypotenuse in any right triangle? - In which In which of the following expressions does the number 16 fill in the blank so that the equation is true? Select all that apply. A) 8(___ + 3) = 32 + 24 B) 8(2 + 9) = ___ + 72 C) 4(7 + 4) = 28 + ___ D) 8(5 + 6) = 40 + ___
- Equations 4648
Solve the system of equations by any method and perform the test: 2 (x + y) -3 (y + 2) = -1 x + 2 / 3y-6 = 2 - Digits A, B, C
For the various digits A, B, and C is true: the square root of the BC is equal to the A, and the sum B+C is equal to A. Calculate A + 2B + 3C. (BC is a two-digit number, not a product). - Star equation
Write digits instead of stars so that the sum of the written digits is odd and is true equality: 42 · ∗8 = 2 ∗∗∗ - Different 55491
Add the same numbers after the same letters and different numbers after the other letters so that equality applies: KRAVA + KRAVA = MLIEKO, where K is an odd digit. - Two numbers
Determine the numbers x and y, so x + y = 8 is true, and the numbers are in the ratio of 4: 5. - Expression 4451
Find the largest natural number d that has that property for any natural number the number n is the value of the expression V (n) = n ^ 4 + 11n²−12 is divisible by d. - Complex number coordinates
Which coordinates show the location of -2+3i - See harmonics
Is it true that the size of the central segment of any trapezoid is the harmonic mean size of its bases? Prove it. The central segment crosses the intersection of the diagonals and is parallel to the bases.
