Isolating a variable in the formula - math word problems - page 39 of 145
Number of problems found: 2883
- Last wagon
 A 200 m long train passes through the 700 m long tunnel so that a time of 1 minute elapses from the entry of the locomotive into the tunnel to the exit of the last wagon from the tunnel. Find the speed of the train.
A 200 m long train passes through the 700 m long tunnel so that a time of 1 minute elapses from the entry of the locomotive into the tunnel to the exit of the last wagon from the tunnel. Find the speed of the train. - Corresponding 59063
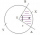 Calculate the radius and area of the circular segment if the center angle = 106° and the length of the corresponding circular arc is l = 52 cm.
Calculate the radius and area of the circular segment if the center angle = 106° and the length of the corresponding circular arc is l = 52 cm. - Circular arc
 Calculate the center angle and length of the circular arc if the radius r = 21 cm and the area of the slice is 328.5 cm²
Calculate the center angle and length of the circular arc if the radius r = 21 cm and the area of the slice is 328.5 cm² - Circular segment
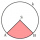 What is the radius of a circular section whose central angle is 36° and the area of S = 53.095 cm²?
What is the radius of a circular section whose central angle is 36° and the area of S = 53.095 cm²? - Circle segment
 A quarter circle with a radius of 4 has the same area as a circle segment with a radius of 3. What is the magnitude of the center angle of the circle segment?
A quarter circle with a radius of 4 has the same area as a circle segment with a radius of 3. What is the magnitude of the center angle of the circle segment? - Two expressions
 When x = 3 and y = 5, how much does the value of 3x² – 2y exceed the value of 2x² – 3y?
When x = 3 and y = 5, how much does the value of 3x² – 2y exceed the value of 2x² – 3y? - ABS triangle
 Point S is the center of the square ABCD. The ABS triangle has an area of 9 cm². What is the circumference of the square in cm?
Point S is the center of the square ABCD. The ABS triangle has an area of 9 cm². What is the circumference of the square in cm? - Isosceles triangle
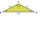 Calculate the height of the isosceles triangle ABC with the base AB, AB = c = 10 cm, and the arms a = b = 13 cm long.
Calculate the height of the isosceles triangle ABC with the base AB, AB = c = 10 cm, and the arms a = b = 13 cm long. - Two forces 3
 Two forces with magnitudes 8 Newtons and 15 Newtons act at a point. Find the angle between the forces if the resultant force is 17 Newtons.
Two forces with magnitudes 8 Newtons and 15 Newtons act at a point. Find the angle between the forces if the resultant force is 17 Newtons. - Calculating 58401
 Suppose the relation s = v applies to the calculation of the path s. t, express the relation for calculating speed v and the relation for calculating time t. Is it true that 10 km will travel at 90 km/h in 10 minutes?
Suppose the relation s = v applies to the calculation of the path s. t, express the relation for calculating speed v and the relation for calculating time t. Is it true that 10 km will travel at 90 km/h in 10 minutes? - 10 workers
 Ten workers will perform the assigned work in 8 days. If six workers work for six days and then another six join them, how long will it take to get the job done?
Ten workers will perform the assigned work in 8 days. If six workers work for six days and then another six join them, how long will it take to get the job done? - Motorist drive
 At what speed did the motorist drive when he reacted by noticing the obstacle by 0.8 s? The magnitude of the opposite acceleration during braking was 6.5 m/s2, and the car ran to a stopped track 35 m.
At what speed did the motorist drive when he reacted by noticing the obstacle by 0.8 s? The magnitude of the opposite acceleration during braking was 6.5 m/s2, and the car ran to a stopped track 35 m. - Which 7
 Which choice is NOT equivalent to the following expression? 9 x { [ 14 ÷ 2 + (4-1) ] - 8 } A 9 x { 10 - 8 } B 9 x { [ 14 ÷ 2 + 3] - 8 } C 9 x 10 - 8 D 18
Which choice is NOT equivalent to the following expression? 9 x { [ 14 ÷ 2 + (4-1) ] - 8 } A 9 x { 10 - 8 } B 9 x { [ 14 ÷ 2 + 3] - 8 } C 9 x 10 - 8 D 18 - Discount
 If someone gives you a discount on "X" at 5% for 60 days. Then the discounted amount is 80000. find the actual amount? Discounted Amount=80000 rate=5% Period=60 days Actual amount=?
If someone gives you a discount on "X" at 5% for 60 days. Then the discounted amount is 80000. find the actual amount? Discounted Amount=80000 rate=5% Period=60 days Actual amount=? - Sides in ratio
 The sides of the triangle are in a ratio of 2:6:5. Find the dimensions of the remaining sides if the longest side is 32 cm.
The sides of the triangle are in a ratio of 2:6:5. Find the dimensions of the remaining sides if the longest side is 32 cm. - Trapezoid and diagonals
 Find the area of a trapezoid with bases a = 24 cm, c = 16 cm, and diagonals u = 22 cm, v = 26 cm.
Find the area of a trapezoid with bases a = 24 cm, c = 16 cm, and diagonals u = 22 cm, v = 26 cm. - The length 8
 The length of a rectangular field is x + 4, and its width is x + 2. What is the area of the field? Answer in polynomials expressions.
The length of a rectangular field is x + 4, and its width is x + 2. What is the area of the field? Answer in polynomials expressions. - Evaluate 19
 Evaluate expression (-8-b)+6c if b=-5 and c=2
Evaluate expression (-8-b)+6c if b=-5 and c=2 - Gale and spruce
 A mighty gale broke the top of the fifteen-year spruce, resting it on the ground. The distance of this top from the trunk was 4.6 m below. At what height was the spruce trunk broken?
A mighty gale broke the top of the fifteen-year spruce, resting it on the ground. The distance of this top from the trunk was 4.6 m below. At what height was the spruce trunk broken? - The block
 The block has dimensions of 5 cm, 10 cm, and 15 cm. Calculate the size of the wall diagonals of this block.
The block has dimensions of 5 cm, 10 cm, and 15 cm. Calculate the size of the wall diagonals of this block.
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
