Spherical segment practice problems
Direction: Solve each problem carefully and show your solution in each item.Number of problems found: 16
- Bracelet 70684
A gold ring with a width of 1 cm is made by drilling a sphere with a radius of 1 cm through its center. A gold bracelet with a width of 1 cm is made by drilling a sphere with a radius of 4 cm through its center. Which piece of jewelry will be worth more i - Portioning ice cream
How many scoops of ice cream can we make using a scoop in the shape of a spherical canopy with a radius of 2.5 cm and a height of 4 cm. We have a 2-liter ice cream tub available. When portioning, we will follow the exact measure. - Sphere cut
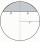A sphere segment is cut off from a sphere k with radius r = 1. The volume of the sphere inscribed in this segment is equal to 1/6 of the segment's volume. What is the distance of the cutting plane from the center of the sphere? - Spherical segment
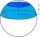Calculate the volume of a spherical segment 18 cm high. The diameter of the lower base is 80 cm, and the upper base is 60 cm.
- Calculate 81034
Calculate the volume of the spherical segment and the surface area of the canopy if the radius of the sphere is r=5cm and the radius of the circular base of the segment ρ=4cm. - Spherical 81527
Sketch a spherical layer formed from a sphere with a radius of r= 8.5cm, given: v=1.5cm, r1=7.7cm, r2=6.8cm. What is its volume? - Spherical segment
The spherical segment with height h=2 has a volume of V=112. Calculate the radius of the sphere which is cut in this segment. - A spherical segment
The aspherical section, whose axial section has an angle of j = 120° in the center of the sphere, is part of a sphere with a radius r = 10 cm. Calculate the cut surface. - Sphere parts, segment
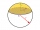A sphere with a diameter of 20.6 cm, the cut is a circle with a diameter of 16.2 cm. What are the volume and surface of the segment?
- Stadium
A domed stadium is shaped like a spherical segment with a base radius of 150 m. The dome must contain a volume of 3500000 m³. Determine the dome's height at its center to the nearest tenth of a meter. - Spherical cap
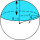Calculate the volume of the spherical cap and the areas of the spherical canopy if r = 5 cm (radius of the sphere), ρ = 4 cm (radius of the circle of the cap). - Paragraphs 69164
What is the volume of the spherical layer that remains after cutting the paragraphs on both sides of the ball, whose height is 3.5 cm? Is the diameter of the ball 24 cm? - Hemispherical hollow
The vessel's hemispherical hollow is filled with water to a height of 10 cm =. How many liters of water are inside if the hollow's inside diameter is d = 28cm? - Hemisphere 70054
Find the volume of the spherical layer that results from a hemisphere with a radius of 5 cm by cutting a paragraph whose height is 1.5 cm.
Calculate the spherical layer's volume that remains from the hemisphere after the 3 cm section is cut. The height of the hemisphere is 10 cm.
Calculate the surface of a spherical paragraph with a height of 6 cm and a radius of 15 cmDo you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
