A spherical segment

A spherical section whose axial section has an angle of j = 120° in the center of the sphere is part of a sphere with a radius r = 10 cm. Calculate the cut surface.

Correct result:

S =  586.2292 cm²

Solution:

$$\begin{gathered} \alpha =120^{\circ } \\ r=10\text{ cm } \\ \sin \alpha \mathrm{/}2=a:r \\ a=r\cdot \sin (\alpha \mathrm{/}2)=10\cdot \sin (120^{\circ }\mathrm{/}2)=8.66025\text{ cm } \\ h=r-\sqrt{r^2-a^2}=10-\sqrt{10^2-8.6603^2}=5\text{ cm } \\ {S}_1=2\pi \cdot r\cdot h=2\cdot 3.1416\cdot 10\cdot 5\doteq 314.1593{\text{cm}}^2 \\ {S}_2=\pi \cdot a\cdot r=3.1416\cdot 8.6603\cdot 10\doteq 272.0699{\text{cm}}^2 \\ S=S_1+S_2=314.1593+272.0699=586.2292{\text{cm}}^2 \end{gathered}$$

Try another example

Showing 0 comments:

Next similar math problems:

• Sphere parts, segment
  A sphere with a diameter of 20.6 cm, the cut is a circle with a diameter of 16.2 cm. .What are the volume of the segment and the surface of the segment?
• Sphere - parts
  Calculate the area of a spherical cap, which is part of an area with base radius ρ = 9 cm and a height v = 3.1 cm.
• Cone A2V
  The surface of the cone in the plane is a circular arc with central angle of 126° and area 415 cm². Calculate the volume of a cone.
• Spherical cap
  The spherical cap has a base radius of 8 cm and a height of 5 cm. Calculate the radius of a sphere of which this spherical cap is cut.
• Spherical section cut
  Find the volume of a spherical section if the radius of its base is 10 cm and the magnitude of the central angle ω = 120 degrees.
• Spherical cap
  Place a part of the sphere on a 4.6 cm cylinder so that the surface of this section is 20 cm². Determine the radius r of the sphere from which the spherical cap was cut.
• Circle sector
  Circular sector with a central angle 160° has area 452 cm². Calculate its radius r.
• Axial section
  The axial section of the cone is an equilateral triangle with area 168 cm². Calculate the volume of the cone.
• Axial cut of a rectangle
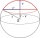  Calculate the volume and surface of the cylinder whose axial cut is a rectangle 15 cm wide with a diagonal of 25 cm long.
• Spherical segment
  The spherical segment with height h=1 has a volume V=223. Calculate the radius of the sphere of which is cut this segment.
• Chord MN
  Chord MN of circle has distance from the center circle S 120 cm. Angle MSN is 64°. Determine the radius of the circle.
• Right triangle trigonometrics
  Calculate the size of the remaining sides and angles of a right triangle ABC if it is given: b = 10 cm; c = 20 cm; angle alpha = 60° and the angle beta = 30° (use the Pythagorean theorem and functions sine, cosine, tangent, cotangent)
• Circular segment
  Calculate the area S of the circular segment and the length of the circular arc l. The height of the circular segment is 2 cm and the angle α = 60°. Help formula: S = 1/2 r2. (Β-sinβ)
• Circle section
  Equilateral triangle with side 33 is inscribed circle section whose center is in one of the vertices of the triangle and the arc touches the opposite side. Calculate: a) the length of the arc b) the ratio betewwn the circumference to the circle sector and
• Cone side
  Calculate the volume and area of the cone whose height is 10 cm and the axial section of the cone has an angle of 30 degrees between height and the cone side.
• Triangle SAS
  Calculate the triangle area and perimeter, if the two sides are 51 cm and 110 cm long and angle them clamped is 130 °.
• Circular pool
  The base of the pool is a circle with a radius r = 10 m, excluding a circular segment that determines the chord length 10 meters. The pool depth is h = 2m. How many hectoliters of water can fit into the pool?