Fraction calculator
This fraction calculator performs basic and advanced fraction operations, expressions with fractions combined with integers, decimals, and mixed numbers. It also shows detailed step-by-step information about the fraction calculation procedure. The calculator helps in finding value from multiple fractions operations. Solve problems with two, three, or more fractions and numbers in one expression.
The result:
11 5/8 + 9 1/2 = 169/8 = 21 1/8 = 21.125
Spelled result in words is one hundred sixty-nine eighths (or twenty-one and one eighth).How do we solve fractions step by step?
- Conversion a mixed number 11 5/8 to a improper fraction: 11 5/8 = 11 5/8 = 11 · 8 + 5/8 = 88 + 5/8 = 93/8
To find a new numerator:
a) Multiply the whole number 11 by the denominator 8. Whole number 11 equally 11 * 8/8 = 88/8
b) Add the answer from the previous step 88 to the numerator 5. New numerator is 88 + 5 = 93
c) Write a previous answer (new numerator 93) over the denominator 8.
Eleven and five eighths is ninety-three eighths. - Conversion a mixed number 9 1/2 to a improper fraction: 9 1/2 = 9 1/2 = 9 · 2 + 1/2 = 18 + 1/2 = 19/2
To find a new numerator:
a) Multiply the whole number 9 by the denominator 2. Whole number 9 equally 9 * 2/2 = 18/2
b) Add the answer from the previous step 18 to the numerator 1. New numerator is 18 + 1 = 19
c) Write a previous answer (new numerator 19) over the denominator 2.
Nine and one half is nineteen halfs. - Add: 93/8 + 19/2 = 93/8 + 19 · 4/2 · 4 = 93/8 + 76/8 = 93 + 76/8 = 169/8
It is suitable to adjust both fractions to a common (equal, identical) denominator for adding, subtracting, and comparing fractions. The common denominator you can calculate as the least common multiple of both denominators - LCM(8, 2) = 8. It is enough to find the common denominator (not necessarily the lowest) by multiplying the denominators: 8 × 2 = 16. In the following intermediate step, it cannot further simplify the fraction result by canceling.
In other words - ninety-three eighths plus nineteen halfs is one hundred sixty-nine eighths.
Rules for expressions with fractions:
Fractions - use a forward slash to divide the numerator by the denominator, i.e., for five-hundredths, enter 5/100. If you use mixed numbers, leave a space between the whole and fraction parts.Mixed numerals (mixed numbers or fractions) keep one space between the integer and
fraction and use a forward slash to input fractions i.e., 1 2/3 . An example of a negative mixed fraction: -5 1/2.
Because slash is both sign for fraction line and division, use a colon (:) as the operator of division fractions i.e., 1/2 : 1/3.
Decimals (decimal numbers) enter with a decimal point . and they are automatically converted to fractions - i.e. 1.45.
Math Symbols
| Symbol | Symbol name | Symbol Meaning | Example |
|---|---|---|---|
| + | plus sign | addition | 1/2 + 1/3 |
| - | minus sign | subtraction | 1 1/2 - 2/3 |
| * | asterisk | multiplication | 2/3 * 3/4 |
| × | times sign | multiplication | 2/3 × 5/6 |
| : | division sign | division | 1/2 : 3 |
| / | division slash | division | 1/3 / 5 |
| : | colon | complex fraction | 1/2 : 1/3 |
| ^ | caret | exponentiation / power | 1/4^3 |
| () | parentheses | calculate expression inside first | -3/5 - (-1/4) |
Examples:
• adding fractions: 2/4 + 3/4• subtracting fractions: 2/3 - 1/2
• multiplying fractions: 7/8 * 3/9
• dividing Fractions: 1/2 : 3/4
• reciprocal of a fraction: 1 : 3/4
• square of a fraction: 2/3^2
• cube of a fraction: 2/3^3
• exponentiation of a fraction: 1/2^4
• fractional exponents: 16 ^ 1/2
• adding fractions and mixed numbers: 8/5 + 6 2/7
• dividing integer and fraction: 5 ÷ 1/2
• complex fractions: 5/8 : 2 2/3
• decimal to fraction: 0.625
• Fraction to Decimal: 1/4
• Fraction to Percent: 1/8 %
• comparing fractions: 1/4 2/3
• multiplying a fraction by a whole number: 6 * 3/4
• square root of a fraction: sqrt(1/16)
• reducing or simplifying the fraction (simplification) : 4/22 - dividing the numerator and denominator of a fraction by the same non-zero number - equivalent fraction
• expression with brackets: 1/3 * (1/2 - 3 3/8)
• compound fraction: 3/4 of 5/7
• fractions multiple: 2/3 of 3/5
• divide to find the quotient: 3/5 ÷ 2/3
The calculator follows well-known rules for the order of operations. The most common mnemonics for remembering this order of operations are:
PEMDAS - Parentheses, Exponents, Multiplication, Division, Addition, Subtraction.
BEDMAS - Brackets, Exponents, Division, Multiplication, Addition, Subtraction
BODMAS - Brackets, Of or Order, Division, Multiplication, Addition, Subtraction.
GEMDAS - Grouping Symbols - brackets (){}, Exponents, Multiplication, Division, Addition, Subtraction.
MDAS - Multiplication and Division have the same precedence over Addition and Subtraction. The MDAS rule is the order of operations part of the PEMDAS rule.
Be careful; always do multiplication and division before addition and subtraction. Some operators (+ and -) and (* and /) have the same priority and must be evaluated from left to right.
Fractions in word problems:
- My whole
 My whole number is 88 if you add 5 thousandths, 8 tenths, and 7 thousandths. What number will I be?
My whole number is 88 if you add 5 thousandths, 8 tenths, and 7 thousandths. What number will I be? - A man 9
 A man earns $2400 in his monthly salary. He spends 3/5 of his salary on food and rent. This month he decided to buy his family presents. What fraction of his money does he spend on presents?
A man earns $2400 in his monthly salary. He spends 3/5 of his salary on food and rent. This month he decided to buy his family presents. What fraction of his money does he spend on presents? - Randy
 Randy solved the following problem: 7/8 + 9/16. He said: I can add 7 and 9 to get 16 and add 8 and 15 to get 23. The answer is 16/23. Is randy correct? Explain.
Randy solved the following problem: 7/8 + 9/16. He said: I can add 7 and 9 to get 16 and add 8 and 15 to get 23. The answer is 16/23. Is randy correct? Explain. - HW store
 At the hardware store, 1/4 of the nails are size 2d, and 1/6 of the nails are size 4d. What fraction of the nails are either size 2d or 4d?
At the hardware store, 1/4 of the nails are size 2d, and 1/6 of the nails are size 4d. What fraction of the nails are either size 2d or 4d? - Work out 2
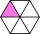 Work out the sum of 2/6 and 1/6. Give your answer in its simplest form.
Work out the sum of 2/6 and 1/6. Give your answer in its simplest form. - There 22
 There is 5/8 of a pizza in one box and 9/12 of a pizza in another box. How much do you have altogether?
There is 5/8 of a pizza in one box and 9/12 of a pizza in another box. How much do you have altogether? - Party pizza
 At a party, there were some pizzas of the same size. Amelia ate 1/3 of a pizza. Chris ate 1/3 of a pizza. Miguel ate 5/12 of a pizza. How many pizzas did the three children eat?
At a party, there were some pizzas of the same size. Amelia ate 1/3 of a pizza. Chris ate 1/3 of a pizza. Miguel ate 5/12 of a pizza. How many pizzas did the three children eat? - Evaluate 27
 Evaluate the expression shown below and write your answer as a fraction in the simplest form. (8)/(3)+ (11)/(12)
Evaluate the expression shown below and write your answer as a fraction in the simplest form. (8)/(3)+ (11)/(12) - Adding two fractions
 Find the missing fraction: 2/5 + 7/10 =
Find the missing fraction: 2/5 + 7/10 = - Evaluate 39
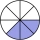 Evaluate the expression shown below and write your answer as a fraction in simplest form. (5)/(12) + (1)/(9) start fraction, 5, divided by, 12, end fraction, plus, one nine.
Evaluate the expression shown below and write your answer as a fraction in simplest form. (5)/(12) + (1)/(9) start fraction, 5, divided by, 12, end fraction, plus, one nine. - Katelyn
 Katelyn ate ⅓ of an apple pie, and Chad ate ⅜ of the same pie. What fraction of the pie was eaten?
Katelyn ate ⅓ of an apple pie, and Chad ate ⅜ of the same pie. What fraction of the pie was eaten? - Add fractions
 Add/subtract the following similar fraction. Express the answer in the lowest terms if possible. Write your answer inside the box: 5/12 + 6/12
Add/subtract the following similar fraction. Express the answer in the lowest terms if possible. Write your answer inside the box: 5/12 + 6/12 - Add two fractions
 What is 1/4 + 10/16?
What is 1/4 + 10/16? - Pizza 4
 Marcus ate half pizza on Monday night. He then ate one-third of the remaining pizza on Tuesday. Which of the following expressions show how much pizza Marcus ate in total?
Marcus ate half pizza on Monday night. He then ate one-third of the remaining pizza on Tuesday. Which of the following expressions show how much pizza Marcus ate in total? - Maximo
 Maximo had 4/6 of a pancake. Kayla gave him another 5/6 of a similar pancake. How many pancakes did Maximo have in the end?
Maximo had 4/6 of a pancake. Kayla gave him another 5/6 of a similar pancake. How many pancakes did Maximo have in the end?
more math problems »