Roof cover

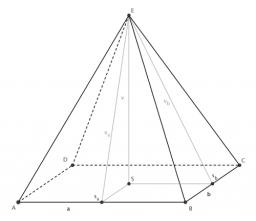
Above the pavilion with a square ground plan with a side length of a = 12 m is a pyramid-shaped roof with a height v = 4.5 m. Calculate how much m² of sheet metal is needed to cover this roof; if 5.5% of the sheet, we must add for joints and waste.

Your answer:

Help us improve! If you spot a mistake, please let let us know. Thank you!

Tips for related online calculators

Our percentage calculator will help you quickly and easily solve a variety of common percentage-related problems.
See also our right triangle calculator.
See also our trigonometric triangle calculator.

You need to know the following knowledge to solve this word math problem:

algebra

rearrange variables

solid geometry

pyramid
surface area

planimetry

Pythagorean theorem
right triangle
area of a shape
triangle
square

basic operations and concepts

percentages

Grade of the word problem

practice for 13 year olds
practice for 14 year olds
high school

We encourage you to watch this tutorial video on this math problem: video1 video2

Related math problems and questions:

Roof sheet calculation
Above the pavilion, with a square floor plan with side a = 12 m, is a pyramid-shaped roof with a height of 4.5 m. How many m² of sheet metal is needed to cover this roof?
Tower
The roof of the tower is a regular hexagonal pyramid with a base edge 5.7 meters long and a height 7 meters. How many m² of the sheet is required to cover the top of the tower? We must add 4% of metal for waste.
The observatory
The dome of the hemisphere-shaped observatory is 5.4 meters high. How many square meters of sheet metal need to be covered to cover it, and must we add 15 percent to the minimum amount due to joints and waste?
Top of the tower
The top of the tower has the shape of a regular hexagonal pyramid. The base edge has a length of 1.2 m. The pyramid height is 1.6 m. How many square meters of sheet metal are needed to cover the top of the tower if 15% extra sheet metal is needed for join
Church roof 2
The roof has the shape of a rotating cone shell with a base diameter of 6 m and a height of 2.5 m. How much money (CZK) will cost the roof cover sheet if 1 m² of metal sheet costs 152 CZK and if you need 15% extra for joints, overlays, and waste?
Pyramid Roof Sheet Metal
The roof of the recreation cottage has the shape of a regular four-sided pyramid with a height of 8 m and a base edge of 4 m. How much ℅ went to folds and joints, and 75.9 square meters of sheet metal were used to cover the roof?
Tent
A pyramid-shaped tent has a base square with a side length of 2 m and a height of 1.7 m. How many meters of canvas is needed to make it if we should add 10% for waste?