Maths practice for 14 year olds - page 46 of 379
Number of problems found: 7575
- Determine 82975
Determine the sum of all positive odd numbers less than 78 - Classmates - girls
Three classmates, Alena, Barbora, and Cecilie, were supposed to divide a certain amount of money. Alena got A Eur, Barbora got B Eur and Cecilie got C Eur. In the division, A: B=9:7 and B: C=6:13 applied. Alena and Cecilie received €1,450 together. How ma - Together 82970
The first tiler would tile the pool inside in 5 working hours; the second would need 7 working hours for the same job. How long would it take them to tile the pool inside together if the second tiler starts working on the pool 12 minutes later than the fi - Minutes 82967
The tank is filled with one inflow in 30 minutes, the other in 24 minutes. How many minutes will it take if both flows are open simultaneously? - Simultaneously 82966
The tank with a volume of 15 hl has two inlets. One flows 5 liters per minute, the other 3 liters per minute. How long will it take for the tank to be filled with both inflows simultaneously? - Together 82965
An older digger would dig a ditch by himself in 6 hours. A younger digger can handle the same trench in 3 hours. How long would it take them to dig if they worked together? - Supporting 82880
Roman and Mathias made a swing in the park by taking a 5 m-long pole and supporting it in the middle. Roman, weighing 52 kg, sat down at the left end. Where should Mathias sit to achieve balance if he weighs 60 kg? Enter the distance measured in meters fr
- Multiplying 82879
By what percent did the product price increase in the store if we proceeded with the price increase by multiplying the original price by 1.16? - Purchased 82877
Mrs. Havelková bought 7 kg of apples and 4 kg of pears for CZK 295. According to the receipt, she found out that 6 kg of apples and 5 kg of pears could be bought at the same cost. Determine the price of 1 kg of purchased apples and 1 kg of purchased pears - Respondents 82860
The students surveyed the number of networks on the Internet. 94% used Facebook, 19% used Twitter, and 42% used Google. How many did not use Facebook if the number of respondents was 310? - Percentage 82856 By what percentage is the number 15 different from the number 12?
- Against 82851
A 3.4 m long ladder is leaning against a wall. Its lower end is 1.6 m away from the wall. At what height does the ladder touch the wall? - Diagonals 82850
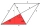How do I find the diagonals of a rhombus if its perimeter is 80dm and one diagonal is 2x larger than the other? - Extraordinary 82849
To the three editors - Brandon, Lubosh, and Lucy - the publishing house owner wants to divide the extraordinary bonus of 1000 euros so that Lubosh gets twice as much as Lucy and Lucy receives three times as much as Brandon. How many euros will each of the - Linnaeus 82847
The cook bought 54 desserts for each of the diners and paid 14.35 Euros for them. Creams were 0.30 Euro each, and Linnaeus cakes were 0.25 Euro each. How many creams and how many Linnaeus cakes did she buy?
- Calculate 82845
Calculate the sum of the first 9 terms of the geometric sequence if a3 equals 2 and a4 equals 8. - Height of poplar
From the 40 m high observation deck, you can see the top of the poplar at a depth angle of 50°10' and the bottom of the poplar at a depth angle of 58°. Calculate the height of the poplar. - Dimensions 82810
The block has 10 cm, 20 cm, and 50 cm dimensions. We reduce the first edge of the cuboid by 20% and increase the second by 20%. How does the volume of the cuboid change? By how many percent? - Cashier 82807 To pay out the amount of CZK 570, the cashier used 15 coins: a few fifty crowns and a few twenty crowns. How did she pay the amount?
- Open simultaneously An empty tank is filled with inflow in 30 minutes. A full tank is emptied in 75 minutes with an open drain. How long does it take for an empty tank to fill up if both the inlet and outlet are open simultaneously?
Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
