Square (second power, quadratic) - math word problems - page 130 of 148
Number of problems found: 2958
- Tiles
 The room has dimensions of 12 m and 5.6 m. Determine the number of square tiles and their largest possible size to cover the room's floor.
The room has dimensions of 12 m and 5.6 m. Determine the number of square tiles and their largest possible size to cover the room's floor. - Tin with oil
 Tin with oil has the shape of a rotating cylinder whose height is equal to the diameter of its base. The canned surface is 1884 cm². Calculate how many liters of oil are in the tin.
Tin with oil has the shape of a rotating cylinder whose height is equal to the diameter of its base. The canned surface is 1884 cm². Calculate how many liters of oil are in the tin. - Rectangular trapezoid
 Calculate the area of a rectangular trapezoid with a right angle at point A and if |AC| = 4 cm, |BC| = 3 cm, and the diagonal AC is perpendicular to the side BC.
Calculate the area of a rectangular trapezoid with a right angle at point A and if |AC| = 4 cm, |BC| = 3 cm, and the diagonal AC is perpendicular to the side BC. - Cellar
 The cellar for storing fruit has a rectangular base with sides of 14 m and 7 meters. You should paint sidewall to 2 m. How many square meters of surface must be painted?
The cellar for storing fruit has a rectangular base with sides of 14 m and 7 meters. You should paint sidewall to 2 m. How many square meters of surface must be painted? - Regular quadrangular pyramid
 How many square meters are needed to cover the shape of a regular quadrangular pyramid base edge of 10 meters if the deviation lateral edges from the base plane are 68°? Calculate waste 10%.
How many square meters are needed to cover the shape of a regular quadrangular pyramid base edge of 10 meters if the deviation lateral edges from the base plane are 68°? Calculate waste 10%. - Ladder 2
 The ladder 9.8 meters long is positioned in the well such that its lower end is distanced from the wall of the well 1.2 m. The upper part of the ladder is supported on the upper edge of the well. How high is the well?
The ladder 9.8 meters long is positioned in the well such that its lower end is distanced from the wall of the well 1.2 m. The upper part of the ladder is supported on the upper edge of the well. How high is the well? - The farmer
 The farmer would like to first seed his small field. The required amount depends on the seed area. The field has a triangular shape. The farmer had a fenced field, so he knew the lengths of the sides: 73, 117, and 63 meters. Find a suitable way to determi
The farmer would like to first seed his small field. The required amount depends on the seed area. The field has a triangular shape. The farmer had a fenced field, so he knew the lengths of the sides: 73, 117, and 63 meters. Find a suitable way to determi - Diamond
 The side length of the diamond is 35 cm, and the length of the diagonal is 56 cm. Calculate the height and length of the second diagonal.
The side length of the diamond is 35 cm, and the length of the diagonal is 56 cm. Calculate the height and length of the second diagonal. - Velocity ratio
 Determine the ratio at which the fluid velocity in different parts of the pipeline (one piece has a diameter of 5 cm and the other has a diameter of 3 cm) when you know that every point of the liquid is the product of the area of the tube [S] and the flui
Determine the ratio at which the fluid velocity in different parts of the pipeline (one piece has a diameter of 5 cm and the other has a diameter of 3 cm) when you know that every point of the liquid is the product of the area of the tube [S] and the flui - Rhombus 2
 Calculate the rhombus area with a height v=48 mm and shorter diagonal u = 60 mm long.
Calculate the rhombus area with a height v=48 mm and shorter diagonal u = 60 mm long. - Cardboard box
 We want to make a cardboard box-shaped quadrangular prism with a rhombic base. The rhombus has a side of 5 cm and 8 cm, one diagonal long. The height of the box is 12 cm. The box will be open at the top. How many square centimeters do we need if we calcul
We want to make a cardboard box-shaped quadrangular prism with a rhombic base. The rhombus has a side of 5 cm and 8 cm, one diagonal long. The height of the box is 12 cm. The box will be open at the top. How many square centimeters do we need if we calcul - Square plate
 The locksmith cut a square metal plate with a sidelong 6 dm into two identical rectangular openings with 2.5 dm and 2 dm dimensions. Calculate the remainder of the square plate.
The locksmith cut a square metal plate with a sidelong 6 dm into two identical rectangular openings with 2.5 dm and 2 dm dimensions. Calculate the remainder of the square plate. - Rhomboid
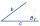 The rhomboid sides' dimensions are a= |AB|=5cm, b = |BC|=6 cm, and the angle's size at vertex A is 60°. What is the length of the diagonal AC?
The rhomboid sides' dimensions are a= |AB|=5cm, b = |BC|=6 cm, and the angle's size at vertex A is 60°. What is the length of the diagonal AC? - Rope slack
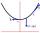 Between two streets, 20 m away, give the lamp in the middle and hang 60 cm below the taut rope. Can it be done with a 20.5 meters rope?
Between two streets, 20 m away, give the lamp in the middle and hang 60 cm below the taut rope. Can it be done with a 20.5 meters rope? - Circumference 1608
 The bases of the isosceles trapezoid measure 110m and 50m. The distance between the bases is 40m. Calculate its circumference.
The bases of the isosceles trapezoid measure 110m and 50m. The distance between the bases is 40m. Calculate its circumference. - Rotary cylinder
 The rotating cylinder has a surface area of 69.08 cm². The area of the shell is 62.8 cm ². What is the diameter of the cylinder?
The rotating cylinder has a surface area of 69.08 cm². The area of the shell is 62.8 cm ². What is the diameter of the cylinder? - Greenhouse
 The Garden plastic greenhouse is shaped like a half-cylinder with a diameter of 6 m and a base length of 20 m. At least how many m² of plastic is needed for its cover?
The Garden plastic greenhouse is shaped like a half-cylinder with a diameter of 6 m and a base length of 20 m. At least how many m² of plastic is needed for its cover? - Water
 The lawn has an area 1571 m², rained 5 mm of water. How many liters of water rained?
The lawn has an area 1571 m², rained 5 mm of water. How many liters of water rained? - Tent
 A pyramid-shaped tent has a base square with a side length of 2 m and a height of 1.7 m. How many meters of canvas is needed to make it if we should add 10% for waste?
A pyramid-shaped tent has a base square with a side length of 2 m and a height of 1.7 m. How many meters of canvas is needed to make it if we should add 10% for waste? - Bottles of juice
 How many 2-liter bottles of juice need to buy if you want to transfer the juice to 50 pitchers' rotary cone shape with a diameter of 24 cm and a base side length of 1.5 dm?
How many 2-liter bottles of juice need to buy if you want to transfer the juice to 50 pitchers' rotary cone shape with a diameter of 24 cm and a base side length of 1.5 dm?
Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
