Extreme 5456
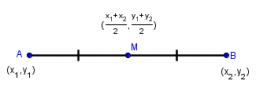
Draw line AB if you know one of its extreme points and the center of line S.
Correct answer:
Tips for related online calculators
You need to know the following knowledge to solve this word math problem:
Themes, topics:
Grade of the word problem:
Related math problems and questions:
- Rhombus construction
Construct ABCD rhombus if its diagonal AC=9 cm and side AB = 6 cm. Inscribe a circle in it, touching all sides. - Department 4220
Draw the line segment AB, AB = 5 cm. Draw a set of points 2 cm away from line AB. What is the district's department? - Connect 6500
Draw the line KL = 55mm. Draw a circle k with center K and a radius of 4cm. Mark the points to belong to the circle and connect them with point L. - Square
Draw a square on the edge of a = 4 cm. Mark the center of symmetry S and all axes of symmetry. How many axes of symmetry do you have? Write down.
- Triangle midpoints
Determine coordinates of triangle ABC vertices if we know triangle sides midpoints SAB [0;3] SBC [1;6] SAC [4;5], its sides AB, BC, AC. - Trisection of a line segment
Divide the line segment AB into three equal parts. Instructions: Construct an equilateral triangle ABC and find its center (e.g., the described circles). - Rhombus construct
Construct parallelogram (rhombus) ABCD, | AB | = 4 cm alpha = 30° and | BD | = 5 cm.
