The areas
The areas of two similar triangles are 12 sq cm and 48 sq cm. If the height of the smaller one is 2.1 cm, then find the height of the bigger one.
Final Answer:
Tips for related online calculators
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
geometryarithmeticplanimetricsUnits of physical quantitiesGrade of the word problem
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- Triangle similarity
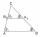Find out if the triangles ABC and A'B'C' are similar, determine the similarity coefficient and write the similarity: a = 40 mm, b = 48 mm, c = 32 mm a´ = 60 mm, b´ = 50 mm, c´ = 40 mm - Rectangular triangles
The lengths of the corresponding sides of two rectangular triangles are in the ratio 2:5. At what ratio are medians relevant to hypotenuse these right triangles? At what ratio are the areas of these triangles? A smaller rectangular triangle has legs 6 and - The sum 42
The sum of two fractions is 6 5/6. If the bigger fraction is subtracted by 3/4, the difference is 4 7/12. What is the smaller fraction? - Parallel sides
Area of trapezium is 45 sq cm and sum of the length of parallel sides is 15 cm. Find height of the trapezium. - Smaller square
Let the points A, B, C and D are midpoints of the sides of the square PQRS. If the area of PQRS is 100 sq cm, what is the area of the smaller square ABCD? - Similarity coefficient
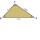The triangles ABC and A'B'C' are similar to the similarity coefficient 2. The sizes of the angles of the triangle ABC are α = 35° and β = 48°. Find the magnitudes of all angles of triangle A'B'C'. - Triangle area percentage
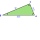A right-angled triangle ABC has sides a = 5 cm, b = 8 cm. The similar triangle A'B'C' is 2.5 times smaller. Calculate the percentage of the area of triangle ABC that is the area of triangle A'B'C'.
