Angle practice problems - page 17 of 63
Number of problems found: 1253
- SSA and geometry
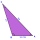The distance between the points P and Q was 356 m measured in the terrain. The viewer can see the PQ line at a 107°22' viewing angle. The observer's distance from P is 271 m. Find the viewing angle of P and the observer. - Clock hands
The hands-on clock shows the time as 12 hours and 2 minutes. Three hours later, calculate the size of an acute angle between the clock hands. - Clock's hands
The clock shows 12 hours. After how many minutes will the angle be between the hour and minute hand 60°? Consider the continuous movement of both hands for hours. - Distance 11711
The observer sits in a room 2 m from a 50 cm wide window. A road runs parallel at a distance of 500 m. What is the cyclist's average speed on this road when the observer sees him at 15 seconds? - Minutes 40411
What path will the end of the second hand make in 24 minutes if it is 5 cm long? - Minutes 6071
What is the angle of the big hand of the watch in 20 minutes? - Clocks
How long will a path of the end of the clock's minute-hand long 23 cm for 340 minutes? How long will we walk at the same time hour-hand? - Rail turn
The curve radius on the railway line is 532 m. The length of the rail track on it is 818 m. How many degrees is the angle ASB if A and B are boundary points and S is the center of the arc curve? - Angle's 7852
The hour and minute hands on the clock face make an alpha angle. If you know it's 10 hours and 12 minutes, what is the angle's size? - Clock Tower
What angle is between the hands-on Clock Tower when it shows 17 hours and 35 minutes? - Traffic laws
Under traffic regulations, car lights can illuminate the road up to a maximum of 30 m. To check the reach of their car's dipped-beam lights, Peter stopped the car 1.5 m from the wall. The dipped-beam headlights are 60 cm high. At what height on the wall d - Juice box 2
The box with juice has the shape of a cuboid. Internal dimensions are 15 cm, 20 cm, and 32 cm. Suppose the box stays at the smallest base juice level and reaches 4 cm below the upper base. How much internal volume of the box fills juice? How many cm below - Describes 82518
The minute hand describes an angle of 180 degrees in half an hour. In how many minutes does the minute hand cover an angle of 192 degrees? - Coal mine
The towing wheel has a diameter of 1.7 meters. How many meters does the elevator cage lower when the wheel turns 32 times? - Concerning 6294
Two isosceles triangles have the same angle at the apex concerning the base. One has a 17 cm long arm and a 10 cm long base. The second has a base length of 8 cm. Determine the length of his arm. - Central angle
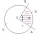The circle has a diameter of 46 cm. What is the arc length that corresponds to a central angle of 30°? - Clock
What distance will pass the end of an 8 cm long hour hand for 15 minutes? - Calculate 7221
Calculate exactly when the hour and minute hands on the clock intersect. - Coin
How many 5-cent coins must be put in a row so that the next 5-cent coins after them (above) are rolled on its axis? - Minutes 13551
What is the angle of the clock hands: a / at 16 hours 15 minutes b / v 8 hours and 4 minutes
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
