Examples for secondary school students - page 26 of 230
Number of problems found: 4591
- Lighting-650W 82356
 In the office will be connected: computer 800 W, kettle 2.5 kW, lighting 650 W, oven 1.9 kW; electric stove 3.3 kW; air conditioning 6100 W. As the smallest cross-section of the cables, we have to bring them to this room. Recalculate for Copper and Alumin
In the office will be connected: computer 800 W, kettle 2.5 kW, lighting 650 W, oven 1.9 kW; electric stove 3.3 kW; air conditioning 6100 W. As the smallest cross-section of the cables, we have to bring them to this room. Recalculate for Copper and Alumin - Determine 82341
 Determine the equation of the circle that is the set of all points of the plane that are twice as far from the point [3,7] as they are from the point [0,1].
Determine the equation of the circle that is the set of all points of the plane that are twice as far from the point [3,7] as they are from the point [0,1]. - Subtract 82333
 I think of three numbers; when I add them, I get 16; when I subtract the third from the sum of the first two numbers, I get 10; when I subtract the second from the sum of the first and third numbers, I get 8. Which numbers do I think?
I think of three numbers; when I add them, I get 16; when I subtract the third from the sum of the first two numbers, I get 10; when I subtract the second from the sum of the first and third numbers, I get 8. Which numbers do I think? - Numbers 82320
 5% of the first and 4% of the second numbers add up to 46. 4% of the first and 5% of the second numbers add up to 44. What are the numbers?
5% of the first and 4% of the second numbers add up to 46. 4% of the first and 5% of the second numbers add up to 44. What are the numbers? - Competition 82310
 In the shooting competition, each shooter fires 5 shots. Shooter Muška will hit the target with one shot with a probability of 60%. What is the probability that he hits at least once out of five shots?
In the shooting competition, each shooter fires 5 shots. Shooter Muška will hit the target with one shot with a probability of 60%. What is the probability that he hits at least once out of five shots? - Boiler 82273
 The ship and boiler are 49 years old. The ship is twice as old as the boiler was when the ship was as old as the boiler is now. How old is the ship?
The ship and boiler are 49 years old. The ship is twice as old as the boiler was when the ship was as old as the boiler is now. How old is the ship? - Accelerates 82218
 The object has a speed of 25m/s and accelerates 5.0m/s² for 250 meters. What is the final velocity?
The object has a speed of 25m/s and accelerates 5.0m/s² for 250 meters. What is the final velocity? - Because of
 Due to the large potato harvest this year, the farm bought a new, more powerful sorter in addition to the old one. Now both machines work simultaneously, and therefore the daily harvest is processed in 12 hours. If only the old machine were working, it wo
Due to the large potato harvest this year, the farm bought a new, more powerful sorter in addition to the old one. Now both machines work simultaneously, and therefore the daily harvest is processed in 12 hours. If only the old machine were working, it wo - Semi-skimmed 82206
 The dairy produces semi-skimmed milk (with 1.5% fat content) by skimming part of the fat from full-fat milk (with 4% fat content). 1 ton of semi-skimmed milk is produced from how many kilograms of fat milk?
The dairy produces semi-skimmed milk (with 1.5% fat content) by skimming part of the fat from full-fat milk (with 4% fat content). 1 ton of semi-skimmed milk is produced from how many kilograms of fat milk? - Individual 82186
 The average height of a member of a family of five is 1.5m, the mode of their height is 1.2m and the median is 1.6m. Find out the heights of individual members of this family if you know that they are integers in dm.
The average height of a member of a family of five is 1.5m, the mode of their height is 1.2m and the median is 1.6m. Find out the heights of individual members of this family if you know that they are integers in dm. - Calculate 82144
 Calculate the height of side b (v_b) of triangle ABC with vertices A[4;1;3] B[2;3;3] and C[1;1;3].
Calculate the height of side b (v_b) of triangle ABC with vertices A[4;1;3] B[2;3;3] and C[1;1;3]. - Distribute 82137
 We have a profit of 40000e, and the work took 30 days. The first worker is promised 38% of the profit but only works for 15 days, the second worker is promised 33% of the profit and works for 20 days, and the third is promised 28% of the profit and works
We have a profit of 40000e, and the work took 30 days. The first worker is promised 38% of the profit but only works for 15 days, the second worker is promised 33% of the profit and works for 20 days, and the third is promised 28% of the profit and works - Parametric 82072
 Convert the parametric expression of the straight line to a general equation. x=3-5t y=-4+10t
Convert the parametric expression of the straight line to a general equation. x=3-5t y=-4+10t - Javelin throwers
 Javelin throwers Anna and Barbora have average throw lengths of 67 m and 75 m, respectively, and standard deviations of 6 m and 3 m, respectively. Assume that the throw lengths have independent normal distributions. Calculate the probability that Anna thr
Javelin throwers Anna and Barbora have average throw lengths of 67 m and 75 m, respectively, and standard deviations of 6 m and 3 m, respectively. Assume that the throw lengths have independent normal distributions. Calculate the probability that Anna thr - Saving problem
 We want to save €25,000. We deposit €3,000 annually, and the interest is 4.4% (interest tax is 19%). How many years do we have to save?
We want to save €25,000. We deposit €3,000 annually, and the interest is 4.4% (interest tax is 19%). How many years do we have to save? - Closest point
 On the line p: 2x + y + 1 = 0, find the point A ∈ p that is closest to the point P =(1,0)
On the line p: 2x + y + 1 = 0, find the point A ∈ p that is closest to the point P =(1,0) - Distribution if the IQ
 Assume that the IQ in the population follows a normal distribution with a mean of 100 points and a standard deviation of 10 points. With what probability among 15 randomly selected people: a. Is there anyone with an IQ above 130 points? b. Are there at le
Assume that the IQ in the population follows a normal distribution with a mean of 100 points and a standard deviation of 10 points. With what probability among 15 randomly selected people: a. Is there anyone with an IQ above 130 points? b. Are there at le - Calculate 82037
 They will calculate the volume of a body that stretches the force gauge in the air with a force of 80N. After immersion in glycerol with a density of 12 kg. m-3 is a load cell stretched by a force of 30N.
They will calculate the volume of a body that stretches the force gauge in the air with a force of 80N. After immersion in glycerol with a density of 12 kg. m-3 is a load cell stretched by a force of 30N. - Determine 82034
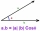 The vectors a = (3, -2), b = (-1, 5) are given. Determine the vector c for which a. c = 17; c = 3
The vectors a = (3, -2), b = (-1, 5) are given. Determine the vector c for which a. c = 17; c = 3 - Principal 82010
 Calculate the interest rate if the principal amount of 500 is 5 euros in interest in 5 months.
Calculate the interest rate if the principal amount of 500 is 5 euros in interest in 5 months.
Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
