Space diagonal - practice problems
Space diagonal (also interior diagonal or body diagonal) of a polyhedron (i.e. prism, cube or rectangular cuboid) is a line connecting two vertices that are not on the same face. Space diagonals contrast with face diagonals, which connect vertices on the same face (but not on the same edge) as each other.Direction: Solve each problem carefully and show your solution in each item.
Number of problems found: 112
- Cuboid - sum of edges length
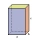Calculate the cuboid's dimensions if the sum of its edges is 19 cm. The body's diagonal size is 13 cm, and its volume is 144 cm³. The total surface area is 192 cm². - Cube in ball
The cube is inscribed into the sphere of radius 181 dm. How many percent is the volume of the cube of the volume of the sphere? - Sphere area
A cube with an edge 1 m long is a circumscribed sphere (vertices of the cube lie on a sphere's surface). Find the surface area of the sphere. - Cube into sphere
The cube has brushed a sphere as large as possible. Determine how much percent the waste was.
- Volume and body diagonal
Calculate how much the cuboid's volume and body diagonal decrease if we reduce each of its three edges, a, b, and c, by 18%. - Billiard balls
A layer of ivory billiard balls radius of 6.35 cm is in the form of a square. The balls are arranged so that each ball is tangent to everyone adjacent to it. In the spaces between sets of 4 adjacent balls, other balls rest, equal in size to the original. - Cubes
One cube is an inscribed sphere, and the other one is described. Calculate the difference of volumes of cubes if the difference of surfaces in 231 cm². - Faces diagonals
Find the cuboid volume if the cuboid's diagonals are x, y, and z (wall diagonals or three faces). Solve for x=1, y=1.1, z=1 - Cuboid diagonal
Calculate the volume and surface area of the cuboid ABCDEFGH, which sides a, b, and c have dimensions in the ratio of 10:8:9. If you know that the diagonal wall AC is 75 cm, and the angle between AC and space diagonal AG is 30 degrees.
- Dimensions 81670
A wooden crate with dimensions d=3m, e=4m, and f=3m was placed in a transport container with dimensions a=10 m, b=4m, and c=3m. What is the maximum length of a straight, rigid rod of negligible diameter that can still be placed in the container in this si - The glass
The glass has the shape of a cylinder with an inner diameter of 12 cm, and the height from the bottom is 16 cm. The cut skewer can be inserted diagonally into the glass so it does not protrude beyond the edge. What is the largest possible length of the cu - Calculate 82567
The volume of a cuboid with a square base is 64 cm3, and the body diagonal deviation from the base's plane is 45 degrees. Calculate its surface area. - Side wall planes
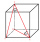Find the volume and surface of a cuboid whose side c is 30 cm long and whose body diagonal forms angles of 24°20' and 45°30' with the planes of the side walls. - Four sided prism
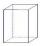Calculate the volume and surface area of a regular quadrangular prism whose height is 28.6cm, and the diagonal body forms a 50-degree angle with the base plane.
- Forces
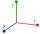In point, G acts three orthogonal forces: F1 = 16 N, F2 = 7 N, and F3 = 6 N. Determine the resultant of F and the angles between F and forces F1, F2, and F3. - Distance of points
A regular quadrilateral pyramid ABCDV is given, in which edge AB = a = 4 cm and height v = 8 cm. Let S be the center of the CV. Find the distance of points A and S. - Calculate cuboid
Given cuboid ABCDEFGH. We know that |AB| = 1 cm, |BC| = 2 cm, |AE| = 3 cm. Calculate in degrees the angle size formed by the lines BG and FH . - Right triangular prism
We have a cuboid with a base and dimensions of 12 cm and 5 cm and a height of 4 cm. The tablecloth is cut into two identical triangular prisms with right triangular bases. We painted the surface of the created prisms with color. Calculate the surface area - Quadrilateral prism
The height of a regular quadrilateral prism is v = 10 cm, and the deviation of the body diagonal from the base is 60°. Determine the length of the base edges, the surface, and the prism's volume.
Calculate the volume (V) and the surface (S) of a regular quadrilateral prism whose height is 28.6 cm and the deviation of the body diagonal from the base plane is 50°.Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
