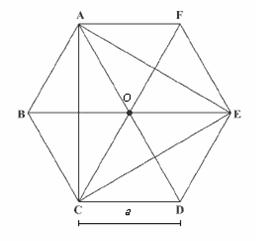
Coordinates hexagon
The regular hexagon ABCDEF is given. Point A has coordinates [1; 3], and point D has coordinates [4; 7]. Calculate the sum of the coordinates of the center of its described circle.
Correct answer:
Tips for related online calculators
You need to know the following knowledge to solve this word math problem:
statisticsgeometryplanimetricsUnits of physical quantitiesGrade of the word problem
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- Chord BC
A circle k has the center at the point S = [0; 0]. Point A = [40; 30] lies on the circle k. How long is the chord BC if the center P of this chord has the coordinates [- 14; 0]? - Difference 80618
A regular hexagon is described and inscribed in a circle. The difference between its areas is 8√3. Find the circle's radius. - Coordinates 36751
Calculate the circle's length and determine the coordinates of the center of the circle when given its diameter XY, X / -3.2 / and Y / -3, -4 /. - Center
In the ABC triangle is point D[1,-2,6], which is the center of the |BC|, and point G[8,1,-3], which is the center of gravity of the triangle. Find the coordinates of the vertex A[x,y,z]. - Circle
From the equation of a circle: -x² -y² +16x -4y -59 = 0 Calculate the coordinates of the center of the circle S[x0, y0] and the radius of the circle r. - Given is
Given is the circle x²+y²-4x+2y-11=0. Calculate the area of the regular hexagon inscribed in the given circle. - Coordinates 83025
Given are points A [1;a2;a3], B [3;-4;-1], C [-3;-1;8]. Points A, B, and C lie in a straight line. Calculate the coordinates a2, a3
