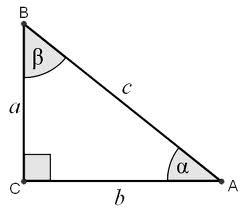
Right-angled 3511
In a right-angled triangle at vertex C, the alpha angle is 24 degrees smaller than the beta angle to determine the size of the triangle angles.
Correct answer:
Tips for related online calculators
Do you have a linear equation or system of equations and are looking for its solution? Or do you have a quadratic equation?
See also our right triangle calculator.
See also our right triangle calculator.
You need to know the following knowledge to solve this word math problem:
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- Remaining 25441
An isosceles triangle has the size of the angles at the base alpha = beta = 34 degrees 34 minutes. Calculate the magnitude of the angle at the remaining vertex of the triangle in degrees and minutes. - Determine 83240
In triangle ABC, the internal angle beta is twice the size of the angle alpha, and the angle gamma is 20 degrees less than the size of the angle beta. Determine the size of all interior angles of this triangle. - Alfa beta gama
The triangle's interior angle beta is 10 degrees greater than the angle alpha, and the gamma angle is three times larger than the beta. Determine the size of the interior angles. - Three angles
In a triangle ABC, the magnitude of the internal angle gamma is equal to one-third of the angle alpha. The size of the angle beta is 80 degrees larger than the size of the gamma angle. Calculate the magnitudes of the interior angles of the triangle ABC.
- Angles of the triangle
ABC is a triangle. The size of the angles alpha and beta are in a ratio of 4:7. The angle gamma is greater than the angle alpha by a quarter of a straight angle. Determine the angles of the triangle ABC. - Alfa, beta, gama
In the ABC triangle, is the size of the internal angle BETA 8 degrees larger than the size of the internal angle ALFA and the size of the internal angle GAMA is twice the size of the angle BETA? Determine the size of the interior angles of the triangle AB - Right-angled 81150
In the right-angled triangle ABC (the right angle at vertex C), the angle ratio is α : β = 5 : 3. Calculate the sizes of these angles and convert them to degrees and minutes (e.g., 45°20')
