Mast
The mast has 16 a long shadow on a slope rising from the mast foot in the direction of the shadow angle at angle 9.7°. Determine the height of the mast if the sun above the horizon is at an angle 40°48'.
Correct answer:
Tips for related online calculators
The line slope calculator is helpful for basic calculations in analytic geometry. The coordinates of two points in the plane calculate slope, normal and parametric line equation(s), slope, directional angle, direction vector, the length of the segment, intersections of the coordinate axes, etc.
Check out our ratio calculator.
Do you want to convert length units?
See also our right triangle calculator.
See also our trigonometric triangle calculator.
Try conversion angle units angle degrees, minutes, seconds, radians, grads.
Check out our ratio calculator.
Do you want to convert length units?
See also our right triangle calculator.
See also our trigonometric triangle calculator.
Try conversion angle units angle degrees, minutes, seconds, radians, grads.
You need to know the following knowledge to solve this word math problem:
- planimetrics
- right triangle
- triangle
- basic functions
- ratio
- reason
- goniometry and trigonometry
- sine
- cosine
- tangent
Units of physical quantities:
Grade of the word problem:
Related math problems and questions:
- Mast shadow
The mast has a 13 m long shadow on a slope rising from the mast foot toward the shadow angle at an angle of 15°. Determine the height of the mast if the sun above the horizon is at an angle of 33°. Use the law of sines. - Vertically 82162
The pole is stuck vertically into the ground. The protruding length is 1m. What is the length of the shadow cast when the sun is just 50° above the horizon? - Mast angles and height
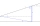Calculate the height of the mast, whose foot can be seen at a depth angle of 11° and the top at a height angle of 28°. The mast is observed from a position 10 m above the level of the base of the mast. - The shadow 2
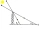The shadow of a tower standing on a level ground is found to be 40 m longer when the Sun’s altitude is 30° than when it is 60°. Find the height of the tower.
- A missile
A missile is fired with a speed of 100 fps in a direction 30° above the horizontal. Determine the maximum height to which it rises. Fps foot per second. - Horizontal 64864
The meter stick is located on the meridian plane and deviated from the horizontal plane to the north by an angle of magnitude 70°. Calculate the length of the shadow cast by a meter stick at true noon if the Sun culminates at an angle of 41°03'. - The mast
We see the top of the pole at an angle of 45°. If we approach the pole by 10 m, we see the top of the pole at an angle of 60°. What is the height of the pole?
