Right triangle + cotangent - practice problems
Number of problems found: 31
- The ladder
 The ladder touches a wall at the height of 7.5 m. The angle of the inclination of the ladder is 76°. How far is the lower end of the ladder from the wall?
The ladder touches a wall at the height of 7.5 m. The angle of the inclination of the ladder is 76°. How far is the lower end of the ladder from the wall? - KLM triangle
 Find the length of the sides of the triangle KLM if m = 5cm height to m = 4.5 cm and size MKL angle is 70 degrees.
Find the length of the sides of the triangle KLM if m = 5cm height to m = 4.5 cm and size MKL angle is 70 degrees. - Building
 How high is the building that throws horizontal shadow 85.6 m long at angle 34°12'?
How high is the building that throws horizontal shadow 85.6 m long at angle 34°12'? - Tree
 Between points A and B is 50m. From A, we see a tree at an angle of 18°. From point B, we see the tree at a three times bigger angle. How tall is a tree?
Between points A and B is 50m. From A, we see a tree at an angle of 18°. From point B, we see the tree at a three times bigger angle. How tall is a tree?
- Depth angles
 At the top of the mountain stands a castle with a tower 30 meters high. We see the crossroad at a depth angle of 32°50' and the heel at 30°10' from the top of the tower. How high is the top of the mountain above the crossroad?
At the top of the mountain stands a castle with a tower 30 meters high. We see the crossroad at a depth angle of 32°50' and the heel at 30°10' from the top of the tower. How high is the top of the mountain above the crossroad? - Traffic sign
 There is a traffic sign for climbing on the road with an angle of 7%. Calculate at what angle the road rises (falls).
There is a traffic sign for climbing on the road with an angle of 7%. Calculate at what angle the road rises (falls). - Right triangle trigonometrics
 Calculate the size of the remaining sides and angles of a right triangle ABC if it is given: b = 10 cm; c = 20 cm; angle alpha = 60°, and the angle beta = 30° (use the Pythagorean theorem and functions sine, cosine, tangent, cotangent)
Calculate the size of the remaining sides and angles of a right triangle ABC if it is given: b = 10 cm; c = 20 cm; angle alpha = 60°, and the angle beta = 30° (use the Pythagorean theorem and functions sine, cosine, tangent, cotangent) - Three pillars
 On a straight road, three pillars are 6 m high at the same distance of 10 m. At what angle of view does Vlado see each pillar if it is 30 m from the first and his eyes are 1.8 m high?
On a straight road, three pillars are 6 m high at the same distance of 10 m. At what angle of view does Vlado see each pillar if it is 30 m from the first and his eyes are 1.8 m high? - Measurements 8129
 The plane flies at an altitude of 22.5 km to the observatory. At the time of the first measurement, it was seen at an elevation angle of 28° and during the second measurement at an elevation angle of 50°. Calculate the distance it flies between these two
The plane flies at an altitude of 22.5 km to the observatory. At the time of the first measurement, it was seen at an elevation angle of 28° and during the second measurement at an elevation angle of 50°. Calculate the distance it flies between these two
- Two boats
 Two boats are located from a height of 150m above the lake's surface at depth angles of 57° and 39°. Find the distance of both boats if the sighting device and both ships are in a plane perpendicular to the lake's surface.
Two boats are located from a height of 150m above the lake's surface at depth angles of 57° and 39°. Find the distance of both boats if the sighting device and both ships are in a plane perpendicular to the lake's surface. - Powerplant chimney
 From the building window at the height of 7.5 m, we can see the top of the factory chimney at an altitude angle of 76° 30 ′. We can see the chimney base from the same place at a 5° 50 ′ depth angle. How tall is the chimney?
From the building window at the height of 7.5 m, we can see the top of the factory chimney at an altitude angle of 76° 30 ′. We can see the chimney base from the same place at a 5° 50 ′ depth angle. How tall is the chimney? - Trigonometric formula
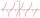 Determine the value of the function tg x (tangent) when cotan x = -0.8 (cotg or cotangent); x holds in the second quadrant)
Determine the value of the function tg x (tangent) when cotan x = -0.8 (cotg or cotangent); x holds in the second quadrant) - A hiker
 A hiker plans to hike up one side of a mountain and down the other side of points a mountain, each side of the mountain formed by a straight line. The angle of elevation at the starting point is 42.4 degrees, and the angle of elevation at the end is 48.3
A hiker plans to hike up one side of a mountain and down the other side of points a mountain, each side of the mountain formed by a straight line. The angle of elevation at the starting point is 42.4 degrees, and the angle of elevation at the end is 48.3 - Perpendicular line
 According to the map, the scouts were supposed to proceed through the forest perpendicular to its straight edge, where the goal was 3 km away from the starting point. They already deviated from the correct direction by 5° at the start. How far from the ta
According to the map, the scouts were supposed to proceed through the forest perpendicular to its straight edge, where the goal was 3 km away from the starting point. They already deviated from the correct direction by 5° at the start. How far from the ta
- Tetrahedral pyramid
 Determine the surface of a regular tetrahedral pyramid when its volume is V = 120 and the angle of the sidewall with the base plane is α = 42° 30'.
Determine the surface of a regular tetrahedral pyramid when its volume is V = 120 and the angle of the sidewall with the base plane is α = 42° 30'. - Fighter
 A military fighter flies at an altitude of 10 km. The ground position was aimed at an altitude angle of 23° and 12 seconds later at an altitude angle of 27°. Calculate the speed of the fighter in km/h.
A military fighter flies at an altitude of 10 km. The ground position was aimed at an altitude angle of 23° and 12 seconds later at an altitude angle of 27°. Calculate the speed of the fighter in km/h. - Cone side
 Calculate the volume and area of the cone whose height is 10 cm, and the axial section of the cone has an angle of 30 degrees between height and the cone side.
Calculate the volume and area of the cone whose height is 10 cm, and the axial section of the cone has an angle of 30 degrees between height and the cone side. - The rescue helicopter
 The rescue helicopter is above the landing site at a height of 180m. The rescue operation site can be seen from here at a depth angle of 52°40'. How far will the helicopter land from the rescue site?
The rescue helicopter is above the landing site at a height of 180m. The rescue operation site can be seen from here at a depth angle of 52°40'. How far will the helicopter land from the rescue site? - Observation tower
 The observation tower has a height of 105 m above sea level. The ship is aimed at a depth angle of 1° 49' from the tower. How far is the ship from the base of the tower?
The observation tower has a height of 105 m above sea level. The ship is aimed at a depth angle of 1° 49' from the tower. How far is the ship from the base of the tower?
Do you have unsolved problem that you need help? Ask a question, and we will try to solve it. Solving math problems.
