Square (second power, quadratic) - math word problems - page 106 of 148

Number of problems found: 2958

Wood lumber
Wooden lumber is 4 m long and has a cross-section square with a side of 15 cm. Calculate: a) the volume of lumber b) the weight of the lumber if 1 m³ weighs 790 kg
Cross-section 4507
How much soil needs to be removed when digging a 200 meter long ditch whose cross-section is an isosceles trapezoid with an area of 4812.5 cm²?
Hamster cage
Ryan keeps his hamster cage on his dresser. The area of the top of Ryan's dresser is 1 2/3 as large as the area of the bottom of his hamster cage. The area of the dresser top is 960 square inches. How many square inches of his dresser top are not covered
Perimeter from area
What is the perimeter of the square if its area is 64 cm²?
Cuboid and eq2
Calculate the volume of a cuboid with a square base and height of 6 cm if the surface area is 48 cm².
Body diagonal
The cuboid has a volume of 32 cm³. Its side surface area is double that of one of the square bases. What is the length of the body diagonal?
Time gone
The Square of Richard's age equals the age of his mother. When he is two times older, his mom will be seven times older than him. Thus, Richard and his mom are seven times older.
Largest number n
Find the largest natural number d that has that property for any natural number n; the number V(n) is the value of the expression: V (n) = n ^ 4 + 11n²−12 is divisible by d.
Resulting 4446
A square with a side length of 3 cm rotates around its diagonal. Calculate the volume and surface area of the resulting body.
Coefficients 4445
Find all triplets P (x) = a * x² + b * x + c with the integer coefficients a, b, and c to which it applies P (1)
Cube and sphere
A cube with a surface area of 150 cm² is described sphere. What is a sphere surface?
Circumference 4421
The area of an equilateral triangle is 81 times three below the square root of cm². Calculate its circumference.
Determine 4414
Determine how many rows the 7th-order square matrix contains.
Third-order 4413
Determine how many elements a third-order square matrix contains.
Cylinder-shaped 4411
A cylinder-shaped hole with a diameter of 12 cm is drilled into a block of height 50 cm with a square base with an edge length of 20 cm. The axis of this opening passes through the center of the base of the cuboid. Calculate the volume and surface area of
Centimeters 4404
Calculate the diagonal of a square if its area is equal to 169 square centimeters.
Rectangular 4402
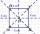Our task is to save the rectangular images with dimensions of 105 mm and 42 mm so that we cover the smallest square. What will be its size, and how many pictures do we need?
Diagonal of square
Calculate the side of a square when its diagonal is 10 cm.
MO-I-Z6
A square with a side of 4 cm is divided into squares with a side of 1 cm as shown in the figure. Divide the square along the marked lines into two figures with a perimeter of 16 cm. Find at least three different solutions (i.e. three such solutions that n
Calculate 4382
Each has a width of 218 cm and an area of 10,900 cm². Calculate the height of the window.

Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.