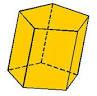
Hexagonal prism 2
The regular hexagonal prism has a surface of 140 cm2 and a height of 5 cm. Calculate its volume.
Your answer:
Your answer:
Tips for related online calculators
Are you looking for help with calculating roots of a quadratic equation?
Do you have a linear equation or system of equations and are looking for a solution? Or do you have a quadratic equation?
Tip: Our volume units converter will help you convert volume units.
See also our trigonometric triangle calculator.
Do you have a linear equation or system of equations and are looking for a solution? Or do you have a quadratic equation?
Tip: Our volume units converter will help you convert volume units.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
algebraarithmeticsolid geometryplanimetryUnits of physical quantitiesGrade of the word problem
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- Hexagonal prism
Calculate the volume and surface of a regular hexagonal prism, the base edge of which is 5 cm long and its height is 20 cm. - Hexagonal prism
Calculate the volume and surface of a regular hexagonal prism with the edge of the base a = 6 cm with the corresponding height v1 = 5.2 cm and the height of the prism h = 1 dm. - Hexagonal prism volume
Calculate the volume and surface of a regular hexagonal prism with a height v = 2 cm and a base edge a = 8 cm. - Hexaprism container Calculate the volume and surface in the shape of a regular hexagonal prism with a height of 1.4 m, a base edge of 3 dm, and a corresponding height of 2.6 dm.
- Circumscribed hexa prism
The regular hexagonal prism is 2 cm high. The radius of the circle circumscribed by the base is 8 cm. Determine its volume and surface. - Pyramid measurements
A regular hexagonal pyramid has a base edge of 20 cm and a lateral edge of 40 cm. Calculate the height and surface area of the pyramid. - The regular
The regular quadrilateral pyramid has a volume of 24 dm³ and a height of 45 cm. Calculate its surface.
