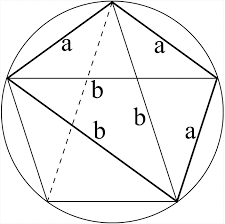
Five-gon

Calculate the side a, the circumference, and the area of the regular 5-angle if Rop = 6cm.

Final Answer:

a =  7.0534 cm
o =  35.2671 cm
S =  85.5951 cm2

Step-by-step explanation:

n=5 R=6 cm  Φ=360/(2⋅ n)=360/(2⋅ 5)=36 ∘  sin Φ = a/2 : R  a=2⋅ R⋅ sinΦ=2⋅ R⋅ sin36° =2⋅ 6⋅ sin36° =2⋅ 6⋅ 0.587785=7.053=7.0534 cm
o=n⋅ a=5⋅ 7.0534=35.2671 cm
h=R2−(a/2)2​=62−(7.0534/2)2​≐4.8541 cm  S1​=2a⋅ h​=27.0534⋅ 4.8541​≐17.119 cm2  S=n⋅ S1​=5⋅ 17.119=85.5951 cm2

Try another example


Did you find an error or inaccuracy? Feel free to send us. Thank you!







Tips for related online calculators
The Pythagorean theorem is the base for the right triangle calculator.
See also our trigonometric triangle calculator.

You need to know the following knowledge to solve this word math problem:

planimetricsUnits of physical quantitiesGrade of the word problem

 
We encourage you to watch this tutorial video on this math problem: video1   video2

Related math problems and questions: