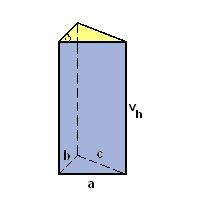
Triangular prism
Calculate the surface area and volume of a three-sided prism with a base of a right-angled triangle if its sides are a = 3 cm, b = 4 cm, c = 5 cm, and the height of the prism is v = 12 cm.
Final Answer:
Tips for related online calculators
See also our right triangle calculator.
Tip: Our volume units converter will help you convert volume units.
See also our trigonometric triangle calculator.
Tip: Our volume units converter will help you convert volume units.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
solid geometryplanimetricsUnits of physical quantitiesGrade of the word problem
Related math problems and questions:
- Right-angled 4951
 Calculate the volume and surface area of the body that is created by cutting out a three-sided prism of the same height from a cuboid with dimensions of 10 cm, 15 cm, and 20 cm, whose base is a right-angled triangle with dimensions of 3 cm, 4 cm, and 5
Calculate the volume and surface area of the body that is created by cutting out a three-sided prism of the same height from a cuboid with dimensions of 10 cm, 15 cm, and 20 cm, whose base is a right-angled triangle with dimensions of 3 cm, 4 cm, and 5 - Right-angled 6034
 A three-sided prism has a base in the shape of a right-angled triangle with a length of 5 cm. The giant wall of the prism shell has a volume of 104 cm². The prism is 8 cm high. Calculate the volume and surface area of the prism.
A three-sided prism has a base in the shape of a right-angled triangle with a length of 5 cm. The giant wall of the prism shell has a volume of 104 cm². The prism is 8 cm high. Calculate the volume and surface area of the prism. - Right-angled triangle base
 Find the volume and surface area of a triangular prism with a right-angled triangle base if the length of the prism base legs are 7.2 cm and 4.7 cm and the height of the prism is 24 cm.
Find the volume and surface area of a triangular prism with a right-angled triangle base if the length of the prism base legs are 7.2 cm and 4.7 cm and the height of the prism is 24 cm. - Triangular RT prism
 Calculate the volume and surface of a triangular prism whose base is a right triangle with sides a = 3m, b = ha = 4m, and c = 5m. The height of the prism is v = 5.5 m.
Calculate the volume and surface of a triangular prism whose base is a right triangle with sides a = 3m, b = ha = 4m, and c = 5m. The height of the prism is v = 5.5 m. - Centimeters 6596
 The jewelry box is in the shape of a four-sided prism with the base of an isosceles trapezoid with sides a=15 centimeters, b is equal to 9 centimeters, c is equal to 10 centimeters, c is equal to 7 whole 4 centimeters. How much fabric is needed to cover a
The jewelry box is in the shape of a four-sided prism with the base of an isosceles trapezoid with sides a=15 centimeters, b is equal to 9 centimeters, c is equal to 10 centimeters, c is equal to 7 whole 4 centimeters. How much fabric is needed to cover a - Perpendicular 35183
 Calculate the surface and volume of a vertical prism if its height h = 18 cm and if the base is an equilateral triangle with side length a = 7.5 cm.
Calculate the surface and volume of a vertical prism if its height h = 18 cm and if the base is an equilateral triangle with side length a = 7.5 cm. - Calculate a prism
 Calculate the volume and surface area of a prism whose height is 16cm and whose base is a right triangle with sides of 5cm and 12cm and a hypotenuse of 13cm.
Calculate the volume and surface area of a prism whose height is 16cm and whose base is a right triangle with sides of 5cm and 12cm and a hypotenuse of 13cm.
