MIT 1869
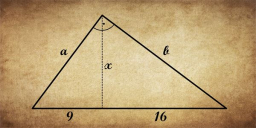
You know the length of parts 9 and 16 of the hypotenuse, at which a right triangle's hypotenuse is divided by a height. The task is to find the lengths of the sides of the triangle and the length of line x. This assignment was part of the Massachusetts Institute of Technology MIT entrance exams in 1869.
Correct answer:
Showing 1 comment:
Peter
Easier solution with almost no calculating. Because all 3 entered triangles are similar, the following holds: x / 9 = 16 / x => x = 12. And because every triangle that has an aspect ratio of 3: 4: 5 is right, it must hold: a = 3 * 5 = 15 ab = 4 * 5 = 20.
PS: Maybe at that time they wanted to know at MIT who was just calculate and who was even thinking.
PS: Maybe at that time they wanted to know at MIT who was just calculate and who was even thinking.
3 years ago 1 Like
Tips for related online calculators
See also our right triangle calculator.
You need to know the following knowledge to solve this word math problem:
Related math problems and questions:
- Height of right RT
The right triangle ABC has a hypotenuse c 9 cm long and a part of the hypotenuse cb = 3 cm. How long is the height of this right triangle? - Sides of right angled triangle
One leg is 1 m shorter than the hypotenuse, and the second leg is 2 m shorter than the hypotenuse. Find the lengths of all sides of the right-angled triangle. - Free space in the garden
The grandfather's free space in the garden was in the shape of a rectangular triangle of 5 meters and 12 meters in length. He decided to divide it into two parts and the height of the hypotenuse. The smaller part creates a rock garden, for the larger sows - Dividing rod
The 3m long rod should be divided into two parts so that one is 16cm longer than the other. Find the lengths of both parts.
- MO - triangles
On the AB and AC sides of the ABC triangle lies successive points E and F, and on segment EF lie point D. The EF and BC lines are parallel. It is true this ratio FD:DE = AE:EB = 2:1. The area of the ABC triangle is 27 hectares, and line segments EF, AD, a - Rectangular triangles
The lengths of the corresponding sides of two rectangular triangles are in the ratio 2:5. At what ratio are medians relevant to hypotenuse these right triangles? At what ratio are the areas of these triangles? A smaller rectangular triangle has legs 6 and - Perpendicular 5516
In a right triangle, one perpendicular is 1 m shorter than the hypotenuse. The other perpendicular is 2 m shorter than the hypotenuse. Find the lengths of all sides of the triangle.
