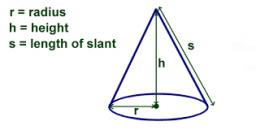
Rotating cone
How does the volume of the rotating cone change if:
a) double the radius of the base
b) We reduce the height three times
c) Reduce the radius of the base five times
Your answer:
a) double the radius of the base
b) We reduce the height three times
c) Reduce the radius of the base five times
Your answer:
Tips for related online calculators
Tip: Our volume units converter will help you convert volume units.
You need to know the following knowledge to solve this word math problem:
solid geometryUnits of physical quantitiesGrade of the word problem
Related math problems and questions:
- Circle area increase
How does the area of a circle change if we double its radius? How many times does its area increase? - Rotating cone
Calculate the volume of a rotating cone with base radius r=18 cm and height h=20 cm. - Prism dimensions
We will double one block dimension and reduce the other by a third. How does its volume change? - Pyramid volume change
How does the volume of a pyramid change if we triple its height? - Sphere radius
We reduce the radius of the sphere by 1/3 of the original radius. How much percent does the volume and surface of the sphere change? - Cone volume surface
The basic parameters of the rotating cone are: Base radius 5 cm The cone height is 12 cm, and the cone side is 13 cm. Calculate: a/volume of the cone b/cone surface - Rotating cone
Calculate the volume and the surface area of a rotating cone of base radius r = 2.3 dm and a height h = 46 mm.
