Right triangle from axes
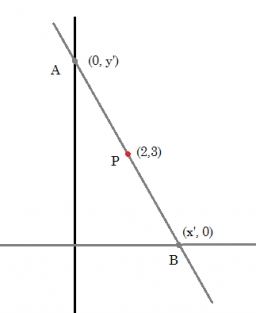
A line segment has its ends on the coordinate axes and forms a triangle of area equal to 36 square units. The segment passes through the point ( 5,2). What is the slope of the line segment?
Correct answer:
Tips for related online calculators
The line slope calculator is helpful for basic calculations in analytic geometry. The coordinates of two points in the plane calculate slope, normal and parametric line equation(s), slope, directional angle, direction vector, the length of the segment, intersections of the coordinate axes, etc.
Are you looking for help with calculating roots of a quadratic equation?
Do you have a linear equation or system of equations and are looking for its solution? Or do you have a quadratic equation?
See also our right triangle calculator.
See also our trigonometric triangle calculator.
Are you looking for help with calculating roots of a quadratic equation?
Do you have a linear equation or system of equations and are looking for its solution? Or do you have a quadratic equation?
See also our right triangle calculator.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
geometryalgebraplanimetricsUnits of physical quantitiesGrade of the word problem
Related math problems and questions:
- Coordinate 82580
Write the equation of the ellipse that passes through the points, and its axes are identical to the coordinate axes when A = [2, 3] and B = [−1, −4]. - Perpendicular 82994
The straight line p is given by the formula y = 1/2 x - 1 . The line q is perpendicular to the line p and passes through the point A [1; 5]. Determine the y-coordinate of the point that intersects the line q with the y-axis. - Direction vector
The line p is given by the point P [- 0,5; 1] and the direction vector s = (1,5; - 3) determines: A) value of parameter t for points X [- 1,5; 3], Y [1; - 2] lines p B) whether the points R [0,5; - 1], S [1,5; 3] lie on the line p C) parametric equations - Line
Line p passes through A[5, -3] and has a direction vector v=(2, 3). Is point B[3, -6] on the line p?
- Slope of line
What is the slope of the line that passes through the points: (-2, 4) and (-3, 1)? - A circle 2
A circle is centered at the point (-7, -1) and passes through the point (8, 7). The radius of the circle is r units. The point (-15, y) lies in this circle. What are r and y (or y1, y2)? - Intersection 81611
Given a triangle ABC: A (-1,3), B(2,-2), C(-4,-3). Determine the coordinates of the intersection of the heights and the coordinates of the intersection of the axes of the sides.
