Centimeters 7406
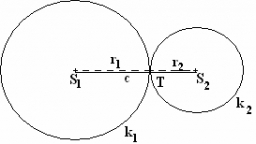
Circles with radii r1 = 10 centimeters and r2 = 4 cm touch from the outside. What is the distance between their centers?
Correct answer:
Tips for related online calculators
Do you want to convert length units?
You need to know the following knowledge to solve this word math problem:
Units of physical quantities:
Grade of the word problem:
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- Intersections 2557
How many intersections do circles with radii of 10 cm and 6 cm have if the distance between their centers is 3 cm? - Circles
For the circle c1(S1; r1=109 cm) and c2(S2; r2 = 152 cm) is distance of centers |S1S2| = 261 cm. Determine the distance between the circles. - Common chord
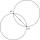Two circles with radii 18 cm and 20 cm intersect at two points. Its common chord is long 11 cm. What is the distance of the centers of these circles? - Intersect 6042
Two circles with straight radii of 58 mm intersect at two points. Their common string is 80 mm long. What is the distance of the centers of these circles?
- Common chord
The common chord of the two circles, c1 and c2, is 3.8 cm long. This chord forms an angle of 47° with the radius r1 in the circle c1. An angle of 24° 30' with the radius r2 is formed in the circle c2. Calculate both radii and the distance between the two - Construct 83156
Construct 2 circles so that their centers are 5 cm apart and: and they had no common touch b- they had a common point They had 2 points in common. - Eq triangle minus arcs
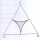In an equilateral triangle with a 2cm long side, the arcs of three circles are drawn from the centers at the vertices and radii 1cm. Calculate the area of the shaded part - a formation that makes up the difference between the triangle area and circular cu
