Area of Right triangle Problems - page 16 of 29
Number of problems found: 564
- Cross-section - trapezoid
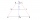The cross-section of the channel has the shape of a trapezoid. The bottom width is 2.25 m, and the depth is 5 m. The walls have a slope of 68°12' and 73°45'. Calculate the upper channel width. - Metal washers
Metal washers with a diameter of 80 mm are cut from a strip of steel sheet with a width of 10 cm and a length of 2 m. When two adjacent circles meet, calculate the material waste percentage if no material is lost. - Isosceles trapezoid
The old father decided to change the top plate of an isosceles-like trapezoid, which has basic dimensions of 120 cm and 60 cm, and a shoulder that is 50 centimeters long. How much does it pay for a new plate and a square meter worth 17 euros? - Flowerbed
The family has tulips on a square flower bed of 6 meters. Later, they added a square terrace with a side of 7 meters to their house. One vertex of the terrace lay exactly in the middle of a tulip bed, and one side of the terrace was divided by the side of - Trapezoid 36701
The field between the two parallel roads is shaped like a trapezoid with 180m and 100m long bases. The distance between the roads is 80m. When the yield of this type of cereal is 8.5 tons from 1 hectare, how many tons of barley were harvested in the field - Isosceles triangle 9
There is an isosceles triangle ABC where AB= AC. The perimeter is 64cm, and the altitude is 24cm. Find the area of the isosceles triangle. - Triangle - many properties
In a right triangle ABC with a right angle at the vertex C, it is given: a = 17cm, Vc = 8 cm. Calculate the length of the sides b, c, its area S, the perimeter o, the length of the radii of the circles of the triangle circumscribed by R and inscribed r an
- Nonagon
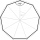Calculate the area and perimeter of a regular nonagon if its radius of the inscribed circle is r = 10cm. - Central park in city
The city park has the shape of a rectangle of 180 meters in length and 120 meters in width. People make their walk through the center of the park from one corner to the second. Calculate how many meters this way is shorter than walking along the path arou - Goat and circles
What is the radius of a circle centered on the other circle, and is the intersection of the two circles equal to half the area of the first circle? This task is the mathematical expression of the role of agriculture. The farmer has circular land on which - Isosceles trapezoid 3
In the isosceles trapezoid ABCD, calculate the unknown side length "a" and its area. Side b = d = 50 cm, c = 20 cm, height = 48 cm. - Gardens
The square garden is 3/4 of the area of the triangular garden, which has sides of 80 m, 50 m, and 50 m. How many meters of fence do we need to fence a square garden? - Chocolate roll
The cube of a 5 cm chocolate roll weighs 30 g. How many calories will the identical chocolate roller of a prism shape with a length of 0.5 m whose cross-section is an isosceles trapezoid with bases 25 and 13 cm and legs 10 cm contain? You know that 100 g - Intersection 81611
Given a triangle ABC: A (-1,3), B(2,-2), C(-4,-3). Determine the coordinates of the intersection of the heights and the coordinates of the intersection of the axes of the sides. - Diagonals at right angle
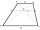In the trapezoid ABCD, this is given: AB=12cm CD=4cm And diagonals crossed under a right angle. What is the area of this trapezoid ABCD?
- A Cartesian framework
1. In a Cartesian framework, the functions f and g we know that: The function (f) is defined by f (x) = 2x², the function (g) is defined by g (x) = x + 3, the point (O) is the origin of the reference, and point (C) is the point of intersection of the grap - Equation of the circle
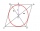Find the equation of the circle inscribed in the rhombus ABCD where A[1, -2], B[8, -3], and C[9, 4]. - Rectangular trapezoid
The ABCD rectangular trapezoid with the AB and CD bases is divided by the diagonal AC into two equilateral rectangular triangles. The length of the diagonal AC is 62cm. Calculate the trapezium area in cm square and calculate how many different perimeters - Equilateral triangle ABC
In the equilateral triangle ABC, K is the center of the AB side, the L point lies on one-third of the BC side near point C, and point M lies on one-third of the side of the AC side closer to point A. Find what part of the ABC triangle contains the triangl - Calculate sides
In the triangle, the side length AB = 6 cm, the height to side c = 5 cm, and the angle BCA = 35°. Calculate sides a b.
Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
Try area of right triangle calculator. See also our right triangle calculator. Examples of area of plane shapes. Right triangle practice problems.
