Diagonal + right triangle - practice problems - page 12 of 18
Number of problems found: 355
- Support colum
Calculate the support column's volume and surface. It is shaped as a vertical quadrangular prism whose base is a rhombus with diagonals u1 = 102 cm and u2 = 64 cm. The column height is 1. 5m. - Flowerbed
The flowerbed has the shape of a truncated pyramid. The bottom edge of the base a = 10 m, and the upper base b = 9 m. The deviation angle between the edge and the base is alpha = 45°. What volume is needed to make this flowerbed? How many plants can be pl - Horizontally 8187
We turn the prism-shaped box with a height of 1 m and a square base with an edge of 0.6 m under a force of 350 N, which acts horizontally compared to the upper edge. What is the weight of the box? - Ratio-cuboid
The lengths of the edges of the cuboid are in the ratio 2:3:6. Its body diagonal is 14 cm long. Calculate the volume and surface area of the cuboid. - Quadrilateral pyramid
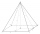The regular quadrilateral pyramid has a base edge a = 1.56 dm and a height h = 2.05 dm. Calculate: a) the deviation angle of the sidewall plane from the base plane b) deviation angle of the side edge from the plane of the base - Surface area of the top
A cylinder is three times as high as it is wide. The length of the cylinder diagonal is 20 cm. Find the exact surface area of the top of the cylinder. - Square pyramid
Calculate the pyramid's volume with the side 5 cm long and with a square base, and the side base has an angle of 60 degrees. - Quadrilateral 16603
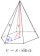Calculate the volume of a regular quadrilateral pyramid, which has the size of the base edge a = 8 cm and the length of the side edge h = 9 cm. - Faces diagonals
Find the cuboid volume if the cuboid's diagonals are x, y, and z (wall diagonals or three faces). Solve for x=1.6, y=1.8, z=1.6 - Body diagonal
Calculate the cube volume, whose body diagonal size is 75 dm. Draw a picture and highlight the body diagonally. - Quadrilateral pyramid
The height of a regular quadrilateral pyramid is 6.5 cm, and the angle between the base and the side wall is 42°. Calculate the surface area and volume of the body—round calculations to 1 decimal place. - Cube diagonals
If you know the length of the body diagonal u = 216 cm, determine the cube's volume and surface area. - Cuboid diagonal
Calculate the volume and surface area of the cuboid ABCDEFGH, which sides a, b, and c have dimensions in the ratio of 10:8:9. If you know that the diagonal wall AC is 75 cm, and the angle between AC and space diagonal AG is 30 degrees. - Quadrilateral 5130
There is a regular quadrilateral pyramid with the base edge length a = 3 cm and with the length of the side edge h = 8 cm. Please calculate its surface area and volume. - Block-shaped 39241
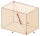The boys wanted to store their hand-made totem 5.1 m high in the block-shaped shed, which measures 4 m, 3 m, and 2 m, for the winter. Will it fit in there at all? - Calculate 32133
The cube has an area of 486 dm². Calculate the length of its side, its volume, the length of the body, and wall diagonals. - Calculate 8354
In a regular pyramid in which the edge of the base is | AB | = 4cm; height = 6cm, calculate the angle of the lines AV and CV, V = vertex. - Diagonal 8192
Find the volume and surface of a cube if you know the length of its body diagonal u = 216 cm. - Axial cut of a rectangle
Calculate the volume and surface of the cylinder whose axial cut is a rectangle 15 cm wide with a diagonal of 25 cm long. - Dimensions 4700
The toolbox has internal dimensions, a length of 1.5 meters, a width of 80 cm, and a height of 6 dm. Calculate the longest rod we can hide in this box.
Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
