Cylinder and Cuboid Volume
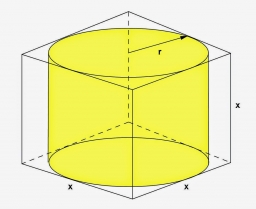
A block with a square base is inserted into a 10-centimeter-high cylinder in such a way that its base is inscribed in the base of the cylinder. The edge of the base of the block measures 4 cm. Both bodies have the same height. Calculate the difference between the volumes of the cylinder and the cuboid
Your answer:
Your answer:
Tips for related online calculators
Tip: Our volume units converter will help you convert volume units.
See also our right triangle calculator.
See also our trigonometric triangle calculator.
See also our right triangle calculator.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
arithmeticsolid geometryplanimetryUnits of physical quantitiesGrade of the word problem
Related math problems and questions:
- Pyramid volume ratio
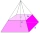A regular quadrilateral pyramid with base edge length a = 15 cm and height v = 21 cm is given. We draw two planes parallel to the base, dividing the height of the pyramid into three equal parts. Calculate the ratio of the volumes of the 3 bodies created. - Cylinder hole
A cylinder-shaped hole with a diameter of 12 cm is drilled into a block of height 50 cm with a square base with an edge length of 20 cm. The axis of this opening passes through the center of the base of the cuboid. Calculate the volume and surface area of - Quadrilateral prism + water
We poured water up to a height of 34 cm into a container of a regular quadrilateral prism with a base edge a = 10.6 cm and a wall diagonal of 3.9 dm. We then inserted a 6 cm long cylinder with a diameter of 10 cm. How many liters of water overflowed? - Cuboid and cube
A block with edges of lengths of 10 cm and 8 cm has the same volume as a cube with an edge of the length of 1 dm. Calculate the third dimension of the block. Compare the ratio of the surfaces of both bodies. - Aquarium
The box-shaped aquarium is 40 cm high; the bottom has 70 cm and 50 cm dimensions. Simon wanted to create an exciting environment for the fish, so he fixed three pillars to the bottom. They all have the shape of a cuboid with a square base. The base edge o - Cone sphere volume
A sphere is inscribed in an equilateral cone with a base diameter of 12 cm. Calculate the volume of both bodies. What percentage of the volume of the cone is filled by the inscribed sphere? - Block cube edge
The block and the cube have the same volumes of 512 cm3, and the block has edge lengths of b = 6.4 cm and c = 10 cm. Can a cube and a block have the same edge length?
