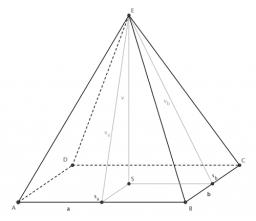
Base diagonal
In a regular four-sided pyramid, the side edge forms an angle of 55° with the base's diagonal. The length of the side edge is eight meters. Calculate the pyramid's surface area and volume.
Your answer:
Your answer:
Tips for related online calculators
Tip: Our volume units converter will help you convert volume units.
See also our right triangle calculator.
See also our trigonometric triangle calculator.
See also our right triangle calculator.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
algebrasolid geometryplanimetrygoniometry and trigonometryUnits of physical quantitiesGrade of the word problem
Related math problems and questions:
- Quadrilateral pyramid
In a regular quadrilateral pyramid, the side edge is e = 7 dm, and the base's diagonal is 50 cm. Calculate the pyramid shell area. - Pyramid Surface Area Ratio
The area of a regular quadrilateral pyramid's mantle is equal to twice its base's area. Calculate the pyramid's surface if the length of the base edge is 20 dm. - Hexagonal pyramid
Calculate a regular hexagonal pyramid's volume and surface area with a base edge a = 30 m and a side edge b = 50 m. - Pyramid - angle
Calculate the regular quadrilateral pyramid's surface, the base edge of which is measured 6 cm, and the deviation from the plane of the base's sidewall plane is 50 degrees. - Pyramid volume calculation The area of a regular quadrilateral pyramid's mantle is equal to twice its base's area. Calculate the pyramid's volume if the base edge's length is 20 dm.
- Four sided prism
Calculate the volume and surface area of a regular quadrilateral prism whose height is 28.6 cm, and the diagonal body forms a 50-degree angle with the base plane. - Side edges
The regular 4-sided pyramid has a body height of 2 dm, and the opposite side edges form an angle of 70°. Calculate the surface area and volume of the pyramid.
