Right triangle practice problems - page 83 of 86
Number of problems found: 1716
- Hexagonal 13891
 A regular hexagonal pyramid has a base inscribed in a circle with a radius of 8 cm and a height of 20 cm. Please sketch the picture. Please calculate the surface of a regular hexagonal pyramid.
A regular hexagonal pyramid has a base inscribed in a circle with a radius of 8 cm and a height of 20 cm. Please sketch the picture. Please calculate the surface of a regular hexagonal pyramid. - Calculate 80636
 Calculate the distance of a chord 19 cm long from the center of a circle with a diameter of 28 cm.
Calculate the distance of a chord 19 cm long from the center of a circle with a diameter of 28 cm. - Parallelogram 54791
 Construct triangle ABC if c = 5cm, b = 7cm and a = 4cm. Then create a parallelogram axially symmetric with the line AC. Measure the size of the second diagonal of this quadrilateral.
Construct triangle ABC if c = 5cm, b = 7cm and a = 4cm. Then create a parallelogram axially symmetric with the line AC. Measure the size of the second diagonal of this quadrilateral. - The quadrilateral pyramid
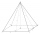 The quadrilateral pyramid has a rectangular base of 24 cm x 3.2dm and a body height of 0.4m. Calculate its volume and surface area.
The quadrilateral pyramid has a rectangular base of 24 cm x 3.2dm and a body height of 0.4m. Calculate its volume and surface area. - Rectangular trapezoid
 The rectangular trapezoid ABCD is: /AB/ = /BC/ = /AC/. The length of the median is 6 cm. Calculate the circumference and area of a trapezoid.
The rectangular trapezoid ABCD is: /AB/ = /BC/ = /AC/. The length of the median is 6 cm. Calculate the circumference and area of a trapezoid. - Pyramid-shaped roof
 A block-shaped shed is covered with a quadrilateral pyramid-shaped roof with a base with sides of 6m and 3m and a height of 2.5m. How many m² (square meters) must be purchased if an extra 40% is calculated for roofing and waste?
A block-shaped shed is covered with a quadrilateral pyramid-shaped roof with a base with sides of 6m and 3m and a height of 2.5m. How many m² (square meters) must be purchased if an extra 40% is calculated for roofing and waste? - Cut and cone
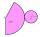 Calculate the volume of the rotation cone whose lateral surface is a circular arc with radius 15 cm and central angle 63 degrees.
Calculate the volume of the rotation cone whose lateral surface is a circular arc with radius 15 cm and central angle 63 degrees. - Polygon 3
 Polygon ABCD is dilated, rotated, and translated to form polygon QWER. The endpoints A and B are at (0, -7) and (8, 8), and the endpoints QW are at (6, -6) and (2, 1.5). What is the scale factor of the dilation?
Polygon ABCD is dilated, rotated, and translated to form polygon QWER. The endpoints A and B are at (0, -7) and (8, 8), and the endpoints QW are at (6, -6) and (2, 1.5). What is the scale factor of the dilation? - Triangular prism
 Calculate the surface of a regular triangular prism; the base's edges are 6 cm long, and the height of the prism is 15 cm.
Calculate the surface of a regular triangular prism; the base's edges are 6 cm long, and the height of the prism is 15 cm. - Quadrilateral pyramid
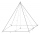 Calculate the surface of a quadrilateral pyramid with a rectangular base, dimensions a = 8 cm, b = 6 cm, and height H = 10 cm.
Calculate the surface of a quadrilateral pyramid with a rectangular base, dimensions a = 8 cm, b = 6 cm, and height H = 10 cm. - Hexagonal 6424
 Calculate the volume and surface of a regular hexagonal prism, the base edge of which is 5 cm long and its height is 20 cm.
Calculate the volume and surface of a regular hexagonal prism, the base edge of which is 5 cm long and its height is 20 cm. - Calculate 2577
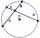 Calculate the length of the circle chord, which is 2.5 cm from the circle's center. The radius is 6.5 cm.
Calculate the length of the circle chord, which is 2.5 cm from the circle's center. The radius is 6.5 cm. - 9-sided pyramid
 Calculate the surface area and volume of a regular nine-sided pyramid if the radius of the circle inscribed in the base measures ρ = 12 cm and the height of the pyramid is 24 cm
Calculate the surface area and volume of a regular nine-sided pyramid if the radius of the circle inscribed in the base measures ρ = 12 cm and the height of the pyramid is 24 cm - Area 4gon
 Calculate the area of 4-gon, two, and the two sides are equal and parallel with lengths 18, 9, 18, and 9. Inner angles are 45°, 135°,45°, 135°.
Calculate the area of 4-gon, two, and the two sides are equal and parallel with lengths 18, 9, 18, and 9. Inner angles are 45°, 135°,45°, 135°. - The tower
 The tower of the Dean's Church in Ústí nad Labem deviates from the original vertical axis by 220 cm. Its original height was 48 m. At what height is the highest point of this tower now? Enter the result to the nearest centimeter.
The tower of the Dean's Church in Ústí nad Labem deviates from the original vertical axis by 220 cm. Its original height was 48 m. At what height is the highest point of this tower now? Enter the result to the nearest centimeter. - Truncated pyramid
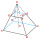 Find the volume and surface area of a regular quadrilateral truncated pyramid if base lengths a1 = 17 cm, a2 = 5 cm, and height v = 8 cm.
Find the volume and surface area of a regular quadrilateral truncated pyramid if base lengths a1 = 17 cm, a2 = 5 cm, and height v = 8 cm. - The cone
 The cone's lateral surface area is 4 cm², and the area of the base is 2 cm². Find the angle in degrees (deviation) of the cone sine and the cone base plane. (The cone side is the segment joining the vertex cone with any point of the base circle. All sides
The cone's lateral surface area is 4 cm², and the area of the base is 2 cm². Find the angle in degrees (deviation) of the cone sine and the cone base plane. (The cone side is the segment joining the vertex cone with any point of the base circle. All sides - Perpendicular sides
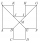 In the ABCDEFGHIJKL, the two adjacent sides are perpendicular to each other, and all sides except the AL and GF sides are identical. The AL and GF sides are twice as long as the other sides. The lines BG and EL intersect at point M. The quadrilateral ABMJ
In the ABCDEFGHIJKL, the two adjacent sides are perpendicular to each other, and all sides except the AL and GF sides are identical. The AL and GF sides are twice as long as the other sides. The lines BG and EL intersect at point M. The quadrilateral ABMJ - Quadrilateral 43941
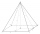 Calculate the surface of a 3.5 m high quadrilateral pyramid with a rectangular base with dimensions of 3 m and 1.8 m.
Calculate the surface of a 3.5 m high quadrilateral pyramid with a rectangular base with dimensions of 3 m and 1.8 m. - Annular area
 The square with side a = 1 is inscribed and circumscribed by circles. Find the annular area.
The square with side a = 1 is inscribed and circumscribed by circles. Find the annular area.
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
