Solid geometry, stereometry - page 100 of 121
Number of problems found: 2404
- Reduce of the volume
Calculate how many % reduce the volume of the cube is reduced the length of each edge by 10%. - Dimensions 44081
In the form of a pyramid on the house with a square floor plan, the roof has dimensions of 12 x 12 m, with a height of 2 m at the highest point. How much roofing do I need to buy? Count on a 10% reserve. - Medal
Calculate the approximate weight of the gold Olympic medal if its diameter is 7 cm and its thickness 6 mm. The density of gold can be found in tables or on the Internet. - Hectoliters - reservoir
The reservoir has the shape of a sphere with a diameter of 10 m. How many hectoliters of water is in it when it is filled to 90%? How many kg of paint are needed for painting if it is painted twice, and 1 kg of paint is enough for 6 square meters? - Whitewash 33351
The castle tower room has the shape of a cylinder with a diameter of 4.6m and a height of 2.9m. Calculate how much it will cost to whitewash the ceiling and walls of this room if €23 is paid for 1 square meter, while windows and doors account for 15℅ of t - Volume of sphere
How many times does the volume of a sphere increase if its radius increases two times? - The ball
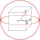The ball has a radius of 2m. What percentage of the surface and volume is another sphere whose radius is 20% larger? - Cross-sections of a cone
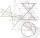Cone with base radius 15 cm and height 20 cm divided by parallel planes to base into three bodies. The planes divide the height of the cone into three equal parts. Determine the volume ratio of the maximum and minimum of the resulting body. - Increases 14471
How many percent will it increase a) surface b) cube volume if the edge of the cube increases by 25%? - Function 8437
Express the volume of a cube as a function of its edge size. - Cardboard - boxes
The closed cardboard box has the shape of a block measuring 25 cm, 1.2 dm, and 0.5 m. How much cardboard is needed to make 20 such boxes? You need to add 5% to bends. - The glass
1 m³ of glass weighs 2600 kg. Calculate the weight of the glass glazing panel with dimensions of 2.5 m and 3.8 m if the thickness of the glass is 0.8 cm. - Roof 8
How many liters of air is under the tower's roof, which has the shape of a regular six-sided pyramid with a 3,6-meter-long bottom edge and a 2,5-meter height? Calculate the supporting columns occupy about 7% of the volume under the roof. - Cylindrical 30331
Calculate the area of sheet metal needed to make a closed cylindrical vessel with a radius of 2.5 m and a height of 1.2 m if the joints and waste count for 6%. - 2x cone
Circular cone height 36 cm was cut plane parallel with the base. The volume of these two small cones is the same. Calculate the height of the smaller cone. - Concrete column
The concrete column of the highway bridge has the shape of a block with dimensions of 1m x 0.8m x 25m. Crane should lift it to a height of 20m. What is the power of his engine if the lifting takes 2 minutes? - Hectoliters - tank
A cylindrical tank with a base diameter of 1.2 m is supposed to hold 17 hectoliters of water. How many square meters of sheet metal are needed to make it? We calculate if 2% of the surfaces are for joints and waste. - Sheet metal troughs
A farmer orders sheet metal troughs from a tinsmith for loading calves on pasture. The tinsmith looks at a picture of the trough and estimates how much sheet metal he will need for one trough. Determine the sheet metal consumption, add 15% for waste and j - Density 6184
What is the weight of the glass door panel if it is 5 mm thick, 2.1 m high, and 6.5 d wide? The density of glass is 2.5 kg/dm³. - Considering 67324
Susan has an old stool - a cube with an edge length of 80 cm. She wants to sew a new cover for her. How many square meters does the fabric consume, considering adding 15% for stitching and folds?
Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
