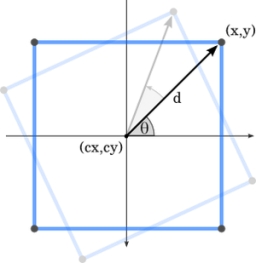
Coordinates of square vertices
I have coordinates of square vertices A / -3; 1/and B/1; 4 /. Find coordinates of vertices C and D, C and D. Thanks, Peter.
Final Answer:
Tips for related online calculators
You need to know the following knowledge to solve this word math problem:
geometryplanimetricsbasic operations and conceptsgoniometry and trigonometryGrade of the word problem
Related math problems and questions:
- Coordinates of square vertices
 The ABCD square has the center S [−3, −2] and the vertex A [1, −3]. Find the coordinates of the other vertices of the square.
The ABCD square has the center S [−3, −2] and the vertex A [1, −3]. Find the coordinates of the other vertices of the square. - Coordinates - rectangle
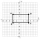 Find the perimeter of the rectangle with vertices A(1,4), B (1,0 ), C (4,0), D (4,4 )
Find the perimeter of the rectangle with vertices A(1,4), B (1,0 ), C (4,0), D (4,4 ) - Determine
 Determine which type of quadrilateral ABCD is and find its perimeter if you know the coordinates of vertices: A/2,4 /, B / -2,1 /, C / -2, -2 /, D/2, -5 /.
Determine which type of quadrilateral ABCD is and find its perimeter if you know the coordinates of vertices: A/2,4 /, B / -2,1 /, C / -2, -2 /, D/2, -5 /. - Three 237
 Three vertices of a rectangle have the coordinates (5, 3), (5, -1), (-1, -1). What are the coordinates of the fourth vertex of the rectangle?
Three vertices of a rectangle have the coordinates (5, 3), (5, -1), (-1, -1). What are the coordinates of the fourth vertex of the rectangle? - The coordinates 4
 The coordinates of the vertices of ∆ABC are respectively (-4, -2), (6, 2) and (4, 6). Find the centroid G of ∆ABC.
The coordinates of the vertices of ∆ABC are respectively (-4, -2), (6, 2) and (4, 6). Find the centroid G of ∆ABC. - The coordinates 3
 The coordinates of two vertices of an equilateral triangle are (1,1) and (5,1). What are the coordinates of the third vertex?
The coordinates of two vertices of an equilateral triangle are (1,1) and (5,1). What are the coordinates of the third vertex? - Polygon - area coordinates
 Find the perimeter and the area of the polygon with the given vertices. T (2,7), U (2,9), V (5,9), W (5,7)
Find the perimeter and the area of the polygon with the given vertices. T (2,7), U (2,9), V (5,9), W (5,7)
