Chord 2

Point A has distance 13 cm from the center of the circle with radius r = 5 cm. Calculate the length of the chord connecting the points T1 and T2 of contact of tangents led from point A to the circle.

Correct result:

x =  9.23 cm

Solution:


Try another example


Our examples were largely sent or created by pupils and students themselves. Therefore, we would be pleased if you could send us any errors you found, spelling mistakes, or rephasing the example. Thank you!


Leave us a comment of this math problem and its solution (i.e. if it is still somewhat unclear...):

Showing 0 comments:
1st comment
Be the first to comment!
avatar




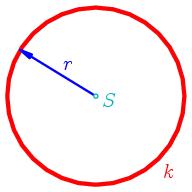
Tips to related online calculators
See also our right triangle calculator.
See also our trigonometric triangle calculator.

You need to know the following knowledge to solve this word math problem:


 
We encourage you to watch this tutorial video on this math problem: video1

Next similar math problems:

  • Chord
    tetiva2_1 It is given to a circle k(r=6 cm) and the points A, B such that / AB / = 8 cm lies on k. Calculate the distance of the center of circle S to the midpoint C of the segment AB.
  • Common chord
    chord2 Two circles with radius 17 cm and 20 cm are intersect at two points. Its common chord is long 27 cm. What is the distance of the centers of these circles?
  • Chord MN
    lyra_tetiva Chord MN of circle has distance from the center circle S 120 cm. Angle MSN is 64°. Determine the radius of the circle.
  • Regular octagon
    220px-N_uholnik Draw the regular octagon ABCDEFGH inscribed with the circle k (S; r = 2.5 cm). Select point S' so that |SS'| = 4.5 cm. Draw S (S '): ABCDEFGH - A'B'C'D'E'F'G'H'.
  • Ace
    esicko The length of segment AB is 24 cm and the point M and N divided it into thirds. Calculate the circumference and area of this shape.
  • Cathethus and the inscribed circle
    RightTriangleInradius In a right triangle is given one cathethus long 14 cm and the radius of the inscribed circle of 5 cm. Calculate the area of this right triangle.
  • Inscribed circle
    vpisana The circle inscribed in a triangle has a radius 3 cm. Express the area of the triangle using a, b, c.
  • Semicircle
    tales-de-mileto In the semicircle with center S and the diameter AB is constructed equilateral triangle SBC. What is the magnitude of the angle ∠SAC?
  • Circles
    three-circles Three circles of radius 95 cm 78 cm and 64 cm is mutually tangent. What is the perimeter of the triangle whose vertices are centers of the circles?
  • Center traverse
    trianles It is true that the middle traverse bisects the triangle?
  • Thales
    circles_1 Calculate the length of the Thales' circle described to right triangle with hypotenuse 18.4 cm.
  • An equilateral
    goat__wolf_and_cabbage_1 An equilateral triangle with a side 10 m represents a wooden platform standing in a lawn. A goat is tied to a corner with a 15 m rope. What is the maximum amount of grazing area available to the goat?
  • Circle inscribed
    inscribed345 Calculate the perimeter and area of a circle inscribed in a triangle measuring 3 , 4 and 5 cm.
  • Right triangle
    triangles_1 Right triangle ABC with side a = 19 and the area S = 95. Calculate the length of the remaining sides.
  • Double ladder
    dvojak The double ladder is 8.5m long. It is built so that its lower ends are 3.5 meters apart. How high does the upper end of the ladder reach?
  • Double ladder
    rr_rebrik The double ladder shoulders should be 3 meters long. What height will the upper top of the ladder reach if the lower ends are 1.8 meters apart?
  • Similarity coefficient
    trig12 The triangles ABC and A "B" C "are similar to the similarity coefficient 2. The sizes of the angles of the triangle ABC are α = 35° and β = 48°. Find the magnitudes of all angles of triangle A "B" C ".