Chord practice problems
A chord of a circle is a straight line segment whose endpoints both lie on the circle. A chord that passes through a circle's center point is the circle's diameter. The word chord is from the Latin chorda meaning bowstring.Instructions: Solve each problem carefully and provide a detailed solution for every item.
Number of problems found: 79
- Three inscribed objects
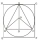A circle is inscribed in a square. An equilateral triangle of side 4√3 is inscribed in that circle. Find the length of the diagonal of the square. - Two chords 2
The length of one of two chords of a circle is 12cm. If the chords are 6cm and 7cm away from the center of the circle, calculate the length of the second chord. - Two chords 6 A chord PQ is 10.4cm long, and its distance from the center of a circle is 3.7cm. Calculate the length of a second chord RS, which is 4.1cm from the center of this circle.
- A chord 2
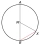A chord of length 16 cm is drawn in a circle of radius 10 cm. Calculate the distance of the chord from the center of the circle. - Chords
How many 5-tónových chords (chord = at the same time sounding different tones) is possible to play within 10 tones? - Endless lego set
The endless Lego set contains only 6, 9, and 20-kilogram blocks that can no longer be polished or broken. The workers took them to the gym and immediately started building different buildings. And, of course, they wrote down how much the building weighed. - Height of the arc - formula
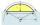Calculate the arc's height if the arc's length is 65 and the chord length is 33. Does there exist a formula to solve this? - Chord of triangle
If the whole chord of the triangle is 14.4 cm long, how do you calculate the shorter and longer parts? - Circle's chords
The circle has two chord lengths, 30 and 34 cm. The shorter one is from the center twice as a longer chord. Determine the radius of the circle. - Circle chord
Determine the circle's radius in which the chord 15 cm away from the center is 21 cm longer than the circle's radius. - Perpendicular diameters
Draw a circle k/S; 4.5 cm/. Next, draw: a/two mutually perpendicular diameters AB and CD b/two radii SA and SE which form an angle of 75 degrees c/chord/KL/= 4 cm d/chord/MN/, which is perpendicular to KL - Chord 5
It is given a circle k/S; 5 cm /. Its chord MN is 3 cm away from the center of the circle. Calculate its length. - Chord 3 The chord is 2/3 of the circle's radius from the center and has a length of 10 cm. How long is the circle radius?
- Chord distance The circle k (S, 6 cm) calculates the chord distance from the center circle S when the chord length is t = 10 cm.
- Circle chord construction
Two line segments of different lengths are given. Construct a circle k so that both line segments are its chords. - Chord
The point on the circle is the endpoint of diameter and endpoint of the chord length of the radius. What angle between chord and diameter? - Distance Between Circle Centers
Two circles with straight radii of 58 mm intersect at two points. Their common string is 80 mm long. What is the distance of the centers of these circles? - Circle
The circle is given by the center on S[-7; 10], and the maximum chord is 13 long. How many intersections have a circle with the coordinate axes? - Common chord
The common chord of the two circles, c1 and c2, is 3.8 cm long. This chord forms an angle of 47° with the radius r1 in the circle c1. An angle of 24° 30' with the radius r2 is formed in the circle c2. Calculate both radii and the distance between the two - Length of the chord
Calculate the length of the chord in a circle with a radius of 25 cm and a central angle of 26°.
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
