Circle chord
Determine the circle's radius in which the chord 15 cm away from the center is 21 cm longer than the circle's radius.
Final Answer:
Tips for related online calculators
Are you looking for help with calculating roots of a quadratic equation?
Do you have a linear equation or system of equations and are looking for its solution? Or do you have a quadratic equation?
The Pythagorean theorem is the base for the right triangle calculator.
Do you want to convert time units like minutes to seconds?
Do you have a linear equation or system of equations and are looking for its solution? Or do you have a quadratic equation?
The Pythagorean theorem is the base for the right triangle calculator.
Do you want to convert time units like minutes to seconds?
You need to know the following knowledge to solve this word math problem:
algebraplanimetricsUnits of physical quantitiesGrade of the word problem
Related math problems and questions:
- Calculate 2577
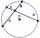Calculate the length of the circle chord, which is 2.5 cm from the circle's center. The radius is 6.5 cm. - Circle chord
Calculate the length of the chord of the circle with radius r = 10 cm, the length of which is equal to the distance from the circle's center. - Circle's chords
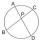The circle has two chord lengths, 30 and 34 cm. The shorter one is from the center twice as a longer chord. Determine the radius of the circle. - Chord 3
The chord is 2/3 of the circle's radius from the center and has a length of 10 cm. How long is the circle radius? - Chord
In a circle with a radius r=60 cm is the chord, 4× longer than its distance from the center. What is the length of the chord? - Calculate the chord
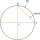The circle's radius is r=8.9 cm, and the chord AB of this circle has a length of 16 cm. Calculate the distance of chord AB from the center of the circle. - Chord 2
Point A has a distance of 13 cm from the circle's center with a radius r = 5 cm. Calculate the length of the chord connecting the points T1 and T2 of contact of tangents led from point A to the circle.
