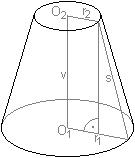
Truncated cone
A truncated cone has bases with 40 cm and 10 cm radiuses and a height of 25 cm. Calculate its surface area and volume.
Your answer:
Your answer:
Tips for related online calculators
Tip: Our volume units converter will help you convert volume units.
You need to know the following knowledge to solve this word math problem:
solid geometryplanimetryUnits of physical quantitiesGrade of the word problem
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- Truncated cone
Calculate the volume of a truncated cone with base radiuses r1=19 cm, r2 = 11 cm, and height v = 5 cm. - Truncated cone and sphere
A sphere is inscribed in a truncated cone with base diameters D1=10 cm and D2=20 cm, touching both bases and the surface. What is its diameter? - Frustrum - volume, area
Calculate the surface and volume of a truncated rotating cone with base radii of 8 cm and 4 cm and a height of 5 cm. - The surface
The surface of a truncated rotating cone with side s = 13 cm is S = 510π cm². Find the radii of the bases when their difference in lengths is 10 cm. - Frustrum - volume, area
Calculate the surface and volume of the truncated cone. The radius of the smaller figure is 4 cm, the height of the cone is 4 cm, and the side of the truncated cone is 5 cm. - Surface of Rotating Cone
The rotating cone has a height of 20 cm and a radius of 18 cm. Calculate its surface. - The truncated The truncated rotating cone has bases with radii r1 = 8 cm, r2 = 4 cm, and height v = 5 cm. What is the volume of the cone from which the truncated cone originated?
