Complexity 30631
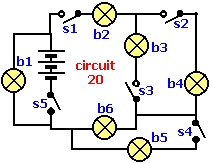
Here, you have a task to think about but don't look for great complexity in it. You have 6 bulbs connected here.
A to F and 6 switches No. 1 to No. 6. Your task will be to gradually determine which bulbs will always be on if any of the switches are in the off position.
A to F and 6 switches No. 1 to No. 6. Your task will be to gradually determine which bulbs will always be on if any of the switches are in the off position.
Correct answer:
Tips for related online calculators
See also our permutations calculator.
See also our variations calculator.
Would you like to compute the count of combinations?
See also our variations calculator.
Would you like to compute the count of combinations?
You need to know the following knowledge to solve this word math problem:
Grade of the word problem:
Related math problems and questions:
- Triangle
Plane coordinates of vertices: K[19, -4] L[9, 13] M[-20, 8] give Triangle KLM. Calculate its area and its interior angles. - An example
An example is playfully for grade 6 from Math, and I don't know how to explain it to my daughter when I don't want to use the calculator to calculate the cube root. Thus: The student made a cuboid from a block of 16x18x48 mm of plasticine. What will be th - Wagons
We have six wagons: two white, two blue, and two red. We assemble trains from them; wagons of the same color are exactly the same, so if we change only two white wagons on a train, it's still the same train because I don't know any difference. How many di - Diagonals at right angle
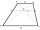In the trapezoid ABCD, this is given: AB=12cm CD=4cm And diagonals crossed under a right angle. What is the area of this trapezoid ABCD?
- Family 8
The father is 38 years old, the daughter is 12, and the son is 14. How many years will a father have as many years as his children together? - Fraction to decimal infinite
Find which digit is at 1000th place after the decimal point in the decimal expansion of the fraction 9/28. - Green - Red
We have 5 bags. Each consists of one green and 2 red balls. From each, we pull just one ball. What is the probability that we don't pull any green ball? - Lidl calculator
We don't like to advertise unnamed retail chains. But offers a relatively high-quality scientific/technical calculator, OLYMPIA 8510S with 97 functions(features) is for 3.48 Eur. Calculate how many cents cost one feature of this calculator. We believe tha - No problem
No problem; here are five decimal addition problems for you to practice: 1. What is the result of 3.5 + 2.1? 2. What is the result of 0.8 + 0.3? 3. What is the result of 5.2 + 1.7? 4. What is the result of 2.5 + 0.9? 5. What is the result of 1.4 + 0.6? I
- Infinity
A square with a side 19 long is an inscribed circle, and the circle is inscribed next square, circle, and so on to infinity. Calculate the sum of the area of all these squares. - Quiz or test
I have a quiz with 20 questions. Each question has four multiple-choice answers, A, B, C, D. THERE IS NO WAY TO KNOW THE CORRECT ANSWER OF ANY GIVEN QUESTION, but the answers are static, in that if the "correct" answer to ; 1 = C, then it will always be e - Inflation 42111
A client deposits 100,000 euros in XYZ bank for a term deposit with an interest rate of 4% pa (per annum). After six months, you went to get the money. How much did he have in his account with the described half-yearly interest? PS When you deposit money - Bivariate data set
Here is a bivariate data set in which you believe y to be the response variable. x y 51.4 44.8 60.2 46.6 60.1 46.8 45.8 46.3 37.4 41.8 72.4 52.5 60 48.2 67.5 49.5 60.9 49.8 51.1 45.7 62.7 49.6 194.9 84.2 Make a scatter plot of this data. Which point is an - Shots
Determine the percentage rate of keeper interventions if 32 shots don't catch four shots.
- News
From 28 students buy magazines, 10 The economist students, 10 Pravda students, and 18 students don't buy any of these magazines. How many students buy both magazines? - Car down
Sarah buys a car costing $12,500. It depreciates value by 8% in the first year, 10% in the second year, and 5% in the third year. Calculate the value of a car after the third year. - Two cars
Two cars started against each other simultaneously to journey long 346 km. The first car went 53 km/h and the second 53 km/h. What distance will be between these cars 20 minutes before a meeting?
