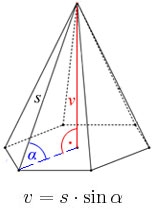
Hexagonal pyramid

Regular hexagonal pyramid has dimensions: length edge of the base a = 1.8 dm and the height of the pyramid = 2.4 dm. Calculate the surface area and volume of a pyramid.

Correct answer:

S =  23.8716 dm²
V =  6.7342 dm³

Step-by-step explanation:

$$\begin{gathered} a=1.8\text{ dm } \\ v=2.4\text{ dm } \\ {h}_1=a\cdot \sqrt{3}\mathrm{/}2=1.8\cdot \sqrt{3}\mathrm{/}2\doteq 1.5588\text{ dm } \\ {h}_2=\sqrt{v^2+h_1^2}=\sqrt{2.4^2+1.5588^2}\doteq 2.8618\text{ dm } \\ {S}_1=a\cdot h_1\mathrm{/}2=1.8\cdot 1.5588\mathrm{/}2\doteq 1.403{\text{dm}}^2 \\ {S}_2=6\cdot S_1=6\cdot 1.403\doteq 8.4178{\text{dm}}^2 \\ {S}_3=a\cdot h_2\mathrm{/}2=1.8\cdot 2.8618\mathrm{/}2\doteq 2.5756{\text{dm}}^2 \\ S=S_2+6\cdot S_3=8.4178+6\cdot 2.5756=23.8716{\text{dm}}^2 \end{gathered}$$

$V={\displaystyle \frac{1}{3}}\cdot S_2\cdot v={\displaystyle \frac{1}{3}}\cdot 8.4178\cdot 2.4=6.7342{\text{dm}}^3$

Try another example

Related math problems and questions:

• Hexagonal pyramid
  Calculate the volume and the surface of a regular hexagonal pyramid with a base edge length of 3 cm and a height of 5 cm.
• Hexagonal pyramid
  Calculate the volume and surface area of a regular hexagonal pyramid with a base edge a = 30 m and a side edge b = 50 m.
• Hexaprism container
  Calculate the volume and surface in the shape of a regular hexagonal prism with a height of 1.4 m with a base edge of 3dm and a corresponding height of 2.6 dm.
• Regular hexagonal pyramid
  Calculate the height of a regular hexagonal pyramid with a base edge of 5 cm and a wall height of w = 20cm. Sketch a picture.
• Hexagon
  Calculate the regular hexagonal prism's surface whose base edge a = 12cm and side edge b = 3 dm.
• Hexagonal pyramid
  Please calculate the height of a regular hexagonal pyramid with a base edge of 5cm and a wall height of w = 20cm. Please sketch a picture.
• Hexagonal pyramid
  Calculate the surface area of a regular hexagonal pyramid with a base inscribed in a circle with a radius of 8 cm and a height of 20 cm.
• Tetrahedral pyramid
  What is the surface of a regular tetrahedral (four-sided) pyramid if the base edge a=16 and height v=16?
• Hexagonal pyramid
  Find the area of a shell of the regular hexagonal pyramid, if you know that its base edge is 5 cm long and the height of this pyramid is 10 cm.
• Hexagonal pyramid
  The base of the pyramid is a regular hexagon, which can be circumscribed in a circle with a radius of 1 meter. Calculate the volume of a pyramid 2.5 meters high.
• The pyramid 4s
  The pyramid with a rectangular base measuring 6 dm and 8 dm has a side edge of length 13 dm. Calculate the surface area and volume of this pyramid.
• Hexagonal pyramid
  Find the volume of a regular hexagonal pyramid, the base edge of which is 12 cm long and the side edge 20 cm.
• Pentagonal pyramid
  The height of a regular pentagonal pyramid is as long as the edge of the base, 20 cm. Calculate the volume and surface area of the pyramid.
• Quadrilateral pyramid
  A regular quadrilateral pyramid has a volume of 24 dm³ and a base edge a = 4 dm. Calculate: a/height of the pyramid b/sidewall height c/surface of the pyramid
• Triangular pyramid
  Calculate the volume and surface area of a regular triangular pyramid with height equal to the base edge 10 cm length.
• 4side pyramid
  Calculate the volume and surface of 4 sides regular pyramid whose base edge is 4 cm long. The angle from the plane of the sidewall and base plane is 60 degrees.
• Base diagonal
  In a regular 4-sided pyramid, the side edge forms an angle of 55° with the base's diagonal. The length of the side edge is eight meters. Calculate the surface area and volume of the pyramid.