The coil
How many ropes (a diameter of 8 mm) fit on the coil (threads are wrapped close together)? The coil has the following dimensions:
The inner diameter is 400mm.
The outside diameter is 800mm.
The length of the coil is 470mm.
The inner diameter is 400mm.
The outside diameter is 800mm.
The length of the coil is 470mm.
Final Answer:

Tips for related online calculators
Do you want to convert area units?
Do you want to convert length units?
Do you know the volume and unit volume, and want to convert volume units?
Do you want to convert length units?
Do you know the volume and unit volume, and want to convert volume units?
You need to know the following knowledge to solve this word math problem:
algebraarithmeticsolid geometryplanimetricsbasic operations and conceptsUnits of physical quantitiesGrade of the word problem
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- Diameter 3962
 The diameter of the atomic nucleus is 10 to -12cm. How many atoms would fit on a 1 mm line if they could be arranged close together?
The diameter of the atomic nucleus is 10 to -12cm. How many atoms would fit on a 1 mm line if they could be arranged close together? - Resistivity 80483
 The heating coil of the cooker has a resistance of 70.5 ohms and is made of a wire with a diameter of 0.30 mm and a length of 9.8 m. Determine the resistivity of the material from which it is made.
The heating coil of the cooker has a resistance of 70.5 ohms and is made of a wire with a diameter of 0.30 mm and a length of 9.8 m. Determine the resistivity of the material from which it is made. - Kitchen
 The kitchen roller has a diameter of 78 mm and a width of 315 mm. How many square millimeters roll on one turn?
The kitchen roller has a diameter of 78 mm and a width of 315 mm. How many square millimeters roll on one turn? - Umbrella
 Can a 75 cm umbrella fit into a box of fruit? The box's dimensions are 390 mm by 510 mm.
Can a 75 cm umbrella fit into a box of fruit? The box's dimensions are 390 mm by 510 mm. - Pipe cross section
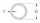 The pipe has an outside diameter of 1100 mm, and the pipe wall is 100 mm thick. Calculate the cross-section of this pipe.
The pipe has an outside diameter of 1100 mm, and the pipe wall is 100 mm thick. Calculate the cross-section of this pipe. - Running
 The length of the inner edge of the running oval is 400 m. The straight sections measure 90 m. Calculate the dimensions of the oval - the rectangle where this oval can fit.
The length of the inner edge of the running oval is 400 m. The straight sections measure 90 m. Calculate the dimensions of the oval - the rectangle where this oval can fit. - Rope
 How many meters of rope 10 mm thick will fit on the bobbin diameter of 200 mm and a length of 350 mm (the central mandrel has a diameter of 50 mm)?
How many meters of rope 10 mm thick will fit on the bobbin diameter of 200 mm and a length of 350 mm (the central mandrel has a diameter of 50 mm)?
