SKMO
Petra had written natural numbers from 1 to 9. She added two of these numbers, deleted them, and wrote the resulting sum instead of the summaries. She thus had eight numbers written down, which she managed to divide into two groups with the same product. Find how large this product could be.
Correct answer:
You need to know the following knowledge to solve this word math problem:
Related math problems and questions:
- Four-digit 10261
Roman likes magic and math. Last time he conjured three- or four-digit numbers like this: • created two new numbers from the given number by dividing it between digits in the place of hundreds and tens (e.g., from the number 581, he would get 5 and 81), • - Double-digit 80970
Eva thought of two natural numbers. She first added these correctly, then subtracted them correctly. In both cases, she got a double-digit result. The product of the resulting two-digit numbers was 645. Which numbers did Eva think of? Please, what is this - Whole numbers
Pavol wrote down a number that is both rational and a whole number. What is one possible number she could have written down? - Remaining 5534
On the table lay eight cards with the numbers 2,3,5,7,11,13,17,19. Fero chose three cards. He added the numbers written on them and found that their sum was 1 more than the sum of the numbers on the remaining cards. Which cards could have been left on the
- Characteristics 2104
Betka thought of a natural number with different digits and wrote it on the board. Podeň wrote the digits of the original number on the back and thus got a new number. By adding these two numbers, he got a number with the same number of digits as the inte - Octahedron - sum
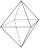On each wall of a regular octahedron is written one of the numbers 1, 2, 3, 4, 5, 6, 7, and 8, wherein on different sides are different numbers. John makes the sum of the numbers written on three adjacent walls for each wall. Thus got eight sums, which al - Decide
The rectangle is divided into seven fields. In each box, write just one of the numbers 1, 2, or 3. Mirek argues that it can be done so that the sum of the two numbers written next to each other is always different. Zuzana (Susan) instead argues that it is
