Distance with Obstacle Measurement
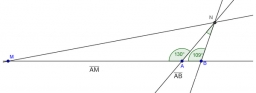
Determine the distance between two places, M, and N, between which there is an obstacle so that place N is not visible from place M. The angles MAN = 130°, NBM = 109°, and the distances |AM| = 54, |BM| = 60, while the points A, B, and M lie on one straight line.
Your answer:
Your answer:
Tips for related online calculators
See also our right triangle calculator.
Cosine rule uses trigonometric SAS triangle calculator.
See also our trigonometric triangle calculator.
Cosine rule uses trigonometric SAS triangle calculator.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
geometryplanimetrygoniometry and trigonometryUnits of physical quantitiesGrade of the word problem
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- Triangulation - 3 places
Determine the distance between two inaccessible places K, L, if the angles KAL=62°10", LAB=41°23", KBL=66°34", and LBA were measured from points A, B, which are 870 m apart = 34°52". Thank you. - Inaccessible direct
Determine the distance between two inaccessible places P, Q, if the distance between two observation points A, B is 2000 m and if you know the size of the angles QAB = 52°40''; PBA = 42°01''; PAB = 86°40'' and QBA = 81°15''. The considered locations A, B, - Angles of elevation
From points A and B on level ground, the angles of elevation of the top of a building are 25° and 37°, respectively. If |AB| = 57 m, calculate, to the nearest meter, the distances of the top of the building from A and B if they are both on the same side o - Point distance marking
In the plane, the points A, B, and C are given 3 cm apart, and they do not lie in the same straight line. Mark the set of all points whose distance from all three points is less than or equal to 2.5 cm. - Radiolocators
An aircraft took off from airport L, is flying in a straight direction at constant altitude, and is being tracked by two radiolocators placed such that their stations A, B and airport L lie in a line. At the first measurement, the aircraft was registered - Unit circle
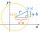In the Cartesian coordinate system, a unit circle is given on which points A and B lie. Point O is the origin with coordinates (0, 0), and point B has coordinates (1, 0). The size of angle BOA is 151°. Determine the x-coordinate of point A. - Tower elevation angles
Find the height of the tower when the geodetic measured two angles of elevation α=34° 30'' and β=41°. The distance between places AB is 14 meters.
