Goniometry and trigonometry - math word problems - page 23 of 32
Number of problems found: 624
- Difference 6029
Between the resorts is 15km, and the climb is 13 permille. What is the height difference? - Straight 21243
The straight railway line has a gradient of 16 permille. What is the size of the pitch angle? - Angle of climb
At what angle does the road rise if the climb is 10%? - The angle of lines
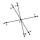Calculate the angle of two lines y=x-8 and y=+12 - Traffic sign
There is a traffic sign for climbing on the road with an angle of 7%. Calculate at what angle the road rises (falls). - Clouds
We see the cloud under an angle of 26°10' and the Sun at an angle of 29°15'. The shade of the cloud is 92 meters away from us. Approximately at what height is the cloud? - Straight road
The straight road has a rise of 19%. How big is the pitch angle? - Depth angles
At the top of the mountain stands a castle with a tower 30 meters high. We see the crossroad at a depth angle of 32°50' and the heel at 30°10' from the top of the tower. How high is the top of the mountain above the crossroad? - Maximum area of rhombus
Calculate the interior angles at which the equilateral rhombus has a maximum area. - Chord 24
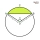A chord with length t = r times the square root of two divides a circle with radius r into two circular segments. What is the ratio of the areas of these segments? - Triangle angles
In the triangle ABC, a: b = 3:2 and α: β = 2:1. Calculate the ratio a: c. - Road
A ratio of 1:15 gives the average climb of the road. By what angle does the road moderate climb? - Wheel gear
A drive wheel of radius two is connected to a drive wheel of radius one by a pulley of length 17. What is the distance between the wheel axles? - Right triangle eq2
Find the lengths of the sides and the angles in the right triangle. Given area S = 210 and perimeter o = 70. - Perimeter - ASA theorem
Calculate the perimeter of the triangle ABC if a = 12 cm, the angle beta is 38 degrees, and the gamma is 92 degrees. - Diamond-air 27221
Find the cut-off angle for the diamond-air pair. n_d = 2.42 α_m =? The absolute refractive index of light for air n = 1 - Sine theorem 2
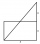From the sine theorem, find the ratio of the sides of a triangle whose angles are 30°, 60°, and 90°. - Right
Determine angles of the right triangle with the hypotenuse c and legs a, b, if: 5a +5b = 7c - Descent of road
The road sign informs us the gradient is 10.9%. Calculate the angle at which the average decreases. - Ratio iso triangle
The ratio of the sides of an isosceles triangle is 7:6:7. Find the base angle to the nearest answer correct to 3 significant figures.
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
