Goniometry and trigonometry - practice problems - page 9 of 32
Number of problems found: 624
- A dodecagon
Find the surface area of a regular 12-sided polygon if its side is a = 12 cm. - Inscribed and described circle
Find the radii of a circle inscribed and circumscribed by a regular pentagon whose side measures 3 cm. - Bevel
I have a bevel in the ratio 1:6. What is the angle, and how do I calculate it? - Base and legs
A right triangle has a base/legs/length of 12 cm, and the angle with the hypotenuse is 13 degrees. What is the length of the second hypotenuse? - In the 18
In the right triangle ABC, The hypotenuse AB = 15 cm, and B = 25 degrees. How long is BC to the nearest centimeter? - Cis notation
Evaluate the multiplication of two complex numbers in cis notation: (6 cis 120°)(4 cis 30°) Write the result in cis and Re-Im notation. - The staircase
The staircase has a total height of 3.6 m and forms an angle of 26° with the horizontal. Calculate the length of the whole staircase. - One side
One side is 36 long with a 15° incline. What is the height at the end of that side? - Dodecagon
Find the area of a regular dodecagon (n=12) if the radius of the circumscribed circle is 5 cm. - Ladder slope
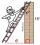What is the slope of a ladder 6.2 m long and 5.12 m in height? - Chimney - view angle
From a distance of 36 meters from the chimney base, its top can be seen at an angle of 53°. Calculate the chimney height and the result round to whole decimeters. - Greatest angle
Calculate the greatest triangle angle with sides 124, 323, 302. - 30-60-90
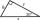The longer leg of a 30°-60°-90° triangle measures 5. What is the length of the shorter leg? - Triangle
Calculate the area of the right triangle ΔABC if one leg is long 12 and its opposite angle is 47°. - Triangle ABC v2 The area of the triangle is 12 cm square. Angle ACB = 30º , AC = (x + 2) cm, BC = x cm. Calculate the value of x.
- Rhomboid
Calculate the circumference and area of the rhomboid with sides 19 and 20, with their angle 40°. - Approaches 45521
The observer lies on the ground at a distance of 20m from a hunting lodge 5m high. A) At what angle of view does the posed see? B) How much does the angle of view change if it approaches the sitting by 5m? - Telegraph poles
The bases of two adjacent telegraph poles have a height difference of 10.5 m. How long do the wires connect the two poles if the slope is 39° 30’? - Circumference 26361
The ABCD diamond has a circumference of 72 cm. The longer diagonal of the animal with the line segment AB angle is 30 °. Calculate the area of the ABCD diamond. - An angle
An angle x is opposite side AB which is 10, and side AC is 15, which is the hypotenuse side in triangle ABC. Calculate angle x.
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
