Pythagorean theorem - math word problems - page 27 of 73
Number of problems found: 1446
- Right triangles
 How many right triangles we can construct from line segments 3,4,5,6,8,10,12,13,15,17 cm long? (Do not forget the triangle inequality).
How many right triangles we can construct from line segments 3,4,5,6,8,10,12,13,15,17 cm long? (Do not forget the triangle inequality). - Height of the arc - formula
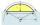 Calculate the arc's height if the arc's length is 65 and the chord length is 33. Does there exist a formula to solve this?
Calculate the arc's height if the arc's length is 65 and the chord length is 33. Does there exist a formula to solve this? - Median
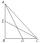 In the ABC triangle is given side a=10 cm and median to side a: ta= 13 cm, and angle gamma 90°. Calculate the length of the median to side b (tb).
In the ABC triangle is given side a=10 cm and median to side a: ta= 13 cm, and angle gamma 90°. Calculate the length of the median to side b (tb). - Isosceles trapezoid
 Calculate the area of an isosceles trapezoid whose bases are in the ratio of 4:3; leg b = 13 cm and height = 12 cm.
Calculate the area of an isosceles trapezoid whose bases are in the ratio of 4:3; leg b = 13 cm and height = 12 cm. - Flowerbed 2
 Around the square flower bed in a park is a sidewalk about 1.3 m wide. The area of this sidewalk is 246 m². What is the area of the flowerbed?
Around the square flower bed in a park is a sidewalk about 1.3 m wide. The area of this sidewalk is 246 m². What is the area of the flowerbed? - Circle section
 An equilateral triangle with side 33 is an inscribed circle section whose center is in one of the triangle's vertices, and the arc touches the opposite side. Calculate: a) the length of the arc b) the ratio between the circumference to the circle sector a
An equilateral triangle with side 33 is an inscribed circle section whose center is in one of the triangle's vertices, and the arc touches the opposite side. Calculate: a) the length of the arc b) the ratio between the circumference to the circle sector a - Calculate 70814
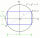 The lengths of the sides AB and AD of the rectangle ABCD are in the ratio 3:4. A circle k with a diameter of 10 cm describes a rectangle. Calculate the side lengths of a given rectangle.
The lengths of the sides AB and AD of the rectangle ABCD are in the ratio 3:4. A circle k with a diameter of 10 cm describes a rectangle. Calculate the side lengths of a given rectangle. - Calculate 39131
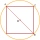 A circle describes a square with a side of 8 cm. Calculate the area of the rest of the circle if we cut out the square.
A circle describes a square with a side of 8 cm. Calculate the area of the rest of the circle if we cut out the square. - Sidewalk 26121
 The garden has a square shape, and its area is 8,100 m². It will be divided by a sidewalk connecting the two opposite garden peaks. How long will this trail be?
The garden has a square shape, and its area is 8,100 m². It will be divided by a sidewalk connecting the two opposite garden peaks. How long will this trail be? - Hexagon 8167
 How many dm² of organic glass is needed to produce 50 washers in the shape of a regular hexagon? The side is 8 cm long.
How many dm² of organic glass is needed to produce 50 washers in the shape of a regular hexagon? The side is 8 cm long. - Square
 Dan's father has a square of 65.25 milligram square of wire with a diagonal. How will the square be big when one mm weighs 7 mg?
Dan's father has a square of 65.25 milligram square of wire with a diagonal. How will the square be big when one mm weighs 7 mg? - Cosine
 Cosine and sine theorem: Calculate all unknown values (sides and angles) of the triangle ABC. a = 20 cm; b = 15 cm; γ = 90°; c =? cm; α =? °; β =? °
Cosine and sine theorem: Calculate all unknown values (sides and angles) of the triangle ABC. a = 20 cm; b = 15 cm; γ = 90°; c =? cm; α =? °; β =? ° - Ratio of squares
 A circle is given, and a square is inscribed. The smaller square is inscribed in a circular arc formed by the square's side and the circle's arc. What is the ratio of the areas of the large and small squares?
A circle is given, and a square is inscribed. The smaller square is inscribed in a circular arc formed by the square's side and the circle's arc. What is the ratio of the areas of the large and small squares? - Irregular pentagon
 A rectangle-shaped, 16 x 4 cm strip of paper is folded lengthwise so that the lower right corner is applied to the upper left corner. What area does the pentagon have?
A rectangle-shaped, 16 x 4 cm strip of paper is folded lengthwise so that the lower right corner is applied to the upper left corner. What area does the pentagon have? - Cable car
 Find the elevation difference of the cable car when it rises by 67 per mille, and the rope length is 930 m.
Find the elevation difference of the cable car when it rises by 67 per mille, and the rope length is 930 m. - Rectangular field
 A rectangular field has a diagonal length of 169m. If the length and width are in the ratio of 12:5. Find the field's dimensions, the field's perimeter, and the field's area.
A rectangular field has a diagonal length of 169m. If the length and width are in the ratio of 12:5. Find the field's dimensions, the field's perimeter, and the field's area. - Diagonals of the rhombus
 How long are the diagonals e, and f in the diamond if its side is 5 cm long and its area is 20 cm²?
How long are the diagonals e, and f in the diamond if its side is 5 cm long and its area is 20 cm²? - Ladder
 Adam placed the ladder of the house, the upper end reaching the window at the height of 3.6m, and the lower end standing on level ground and distant from a wall of 1.5m. What is the length of the ladder?
Adam placed the ladder of the house, the upper end reaching the window at the height of 3.6m, and the lower end standing on level ground and distant from a wall of 1.5m. What is the length of the ladder? - Surveyor
 Calculate the area of what may vary rectangular if it was focused by a surveyor and found the dimensions 10 x 16 m while in each of the four joint points can be position deviation 8 cm?
Calculate the area of what may vary rectangular if it was focused by a surveyor and found the dimensions 10 x 16 m while in each of the four joint points can be position deviation 8 cm? - Rhombus
 It is given a rhombus with a side length of a = 20 cm. Touchpoints of the inscribed circle divided its sides into sections a1 = 13 cm and a2 = 7 cm. Calculate the radius r of the circle and the length of the diagonals of the rhombus.
It is given a rhombus with a side length of a = 20 cm. Touchpoints of the inscribed circle divided its sides into sections a1 = 13 cm and a2 = 7 cm. Calculate the radius r of the circle and the length of the diagonals of the rhombus.
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
