Octagon perimeter area
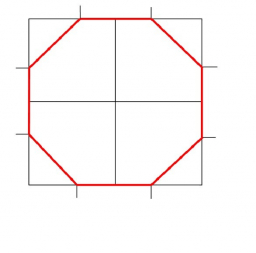
From a square with a side of 4 cm, we cut four right-angled isosceles triangles with right angles at the square's vertices and with an overlap of √2 cm. We get an octagon. Calculate its perimeter if the area of the octagon is 14 cm².
Your answer:
Your answer:
Tips for related online calculators
See also our right triangle calculator.
Tip: Our volume units converter will help you convert volume units.
Tip: Our volume units converter will help you convert volume units.
You need to know the following knowledge to solve this word math problem:
arithmeticplanimetryUnits of physical quantitiesGrade of the word problem
Related math problems and questions:
- Board triangle ratio
From a rectangular board with 2 m and 3 m dimensions, we cut isosceles and right-angled triangles at the corners with an overhang of 40 cm. Calculate the ratio of the rest of the board's areas to its total original area. - Octagon from rectangle
We cut the corners of a rectangular tablecloth with dimensions of 4 dm and 8 dm into isosceles triangles. Thus, the octagon formed had an area of 26 dm². How many dm² of tablecloth do we cut down? - Triangle calculations
a) The perimeter of the equilateral triangle ABC is 63 cm. Calculate the side sizes of the triangle and its height. b) A right isosceles triangle has an area of 40.5 square meters. How big is his circuit? c) Calculate the square's area if the diagonal's s - Recursion squares
In the square, ABCD has inscribed a square so that its vertices lie at the centers of the sides of the square ABCD. The procedure of inscribing the square is repeated this way. The side length of the square ABCD is a = 20 cm. Calculate: a) the sum of peri - Candy - MO
Gretel deploys different numbers to the vertex of a regular octagon, from one to eight candy. Peter can then choose which three piles of candy to give Gretel others retain. The only requirement is that the three piles lie at the vertices of an isosceles t - Right Triangle Perimeter
The side lengths of two triangles, sorted by size, are: 8 cm, 10 cm, 13 cm, 15 cm, 17 cm, and 19 cm. One of these two triangles is right-angled. Calculate the perimeter of this right triangle in centimeters - Similarity of squares
There is a given square ABCD with a = 5.3 cm. If the similarity ratio k = 3 cm, determine the side size of a similar square. Then, calculate the area and perimeter of the magnified square.
